一、单选题 (共 38 题,每小题 5 分,共 50 分,每题只有一个选项正确)
函数 $f(x)=\frac{1}{3} x^3+\frac{1}{2} x^2+6 x+1$ 的图形在点 $(0,1)$ 处的切线与 $x$ 轴交点的坐标是
$\text{A.}$ $\left(-\frac{1}{6}, 0\right)$
$\text{B.}$ $(-1,0)$
$\text{C.}$ $\left(\frac{1}{6}, 0\right)$
$\text{D.}$ $(1,0)$
若 $-3 a^2-5 b < 0$ ,则方程 $x^5+2 a x^3+3 b x+4 c=0$
$\text{A.}$ 无实根
$\text{B.}$ 有唯一实根
$\text{C.}$ 有三个不同实根
$\text{D.}$ 有五个不同实根
若 $y=x^2+a x+b$ 和 $2 y=-1+x y^3$ 在 $(1,-1)$ 点相切,其中 $a, b$ 是常数,则
$\text{A.}$ $a=0, b=-2$
$\text{B.}$ $a=1, b=-3$
$\text{C.}$ $a=-3, b=1$
$\text{D.}$ $a=-1, b=-1$
如图, $x$ 轴上有一线密度为常数 $\mu$ ,长度为 $l$ 的细杆,若质量为 $m$ 的质点到杆右端的距离为 $a$ ,已知引力系数为 $k$ ,则质点和细杆之间引力的大小为
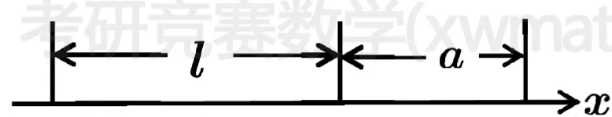
$\text{A.}$ $\int_{-l}^0 \frac{k m \mu \mathrm{d} x}{(a-x)^2}$
$\text{B.}$ $\int_0^l \frac{k m \mu \mathrm{d} x}{(a-x)^2}$
$\text{C.}$ $2 \int_{-\frac{l}{2}}^0 \frac{k m \mu \mathrm{d} x}{(a+x)^2}$
$\text{D.}$ $2 \int_0^{\frac{l}{2}} \frac{k m \mu \mathrm{d} x}{(a+x)^2}$
设函数 $z=f(x, y)$ 的全微分为 $\mathrm{d} z=x \mathrm{~d} x+y \mathrm{~d} y$ ,则点 $(0,0)$
$\text{A.}$ 不是 $f(x, y)$ 的连续点
$\text{B.}$ 不是 $f(x, y)$ 的极值点
$\text{C.}$ 是 $f(x, y)$ 的极大值点
$\text{D.}$ 是 $f(x, y)$ 的极小值点
使不等式 $\int_1^x \frac{\sin t}{t} \mathrm{~d} t>\ln x$ 成立的 $x$ 的范围是
$\text{A.}$ $(0,1)$
$\text{B.}$ $\left(1, \frac{\pi}{2}\right)$
$\text{C.}$ $\left(\frac{\pi}{2}, \pi\right)$
$\text{D.}$ $(\pi,+\infty)$
曲线 $y=x^2$ 与曲线 $y=a \ln x(a \neq 0)$ 相切, 则
$\text{A.}$ $4 e$
$\text{B.}$ $3 e$
$\text{C.}$ $2 e$
$\text{D.}$ $ e$
设函数 $f(x), g(x)$ 具有二阶导数,且 $g^{\prime \prime}(x) < 0$ ,若 $g\left(x_0\right)=a$ 是 $g(x)$ 的极值,则 $f(g(x))$ 在 $x_0$ 取极大值的一个充分条件是
$\text{A.}$ $f^{\prime}(a) < 0$
$\text{B.}$ $f^{\prime}(a)>0$
$\text{C.}$ f $^{\prime \prime}(a) < 0$
$\text{D.}$ $f^{\prime \prime}(a)>0$
设函数 $f(x)$ 具有二阶连续导数,且 $f(x)>0 , f^{\prime}(0)=0$ ,则函数 $z=f(x) \ln f(y)$ 在点 $(0,0)$ 处取得极小值的一个充分条件是
$\text{A.}$ $f(0)>1, f^{\prime \prime}(0)>0$
$\text{B.}$ $f(0)>1, \quad f^{\prime \prime}(0) < 0$
$\text{C.}$ $f(0) < 1, f^{\prime \prime}(0)>0$
$\text{D.}$ $f(0) < 1, \quad f^{\prime \prime}(0) < 0$
设函数 $f(x), g(x)$ 均有二阶连续导数,满足 $f(0)>0, g(0) < 0$ 且 $f^{\prime}(0)=g^{\prime}(0)=0$ ,则函数 $z=f(x) g(y)$ 在点 $(0,0)$ 处取得极小值的一个充分条件是
$\text{A.}$ $f^{\prime \prime}(0) < 0, g^{\prime \prime}(0)>0$
$\text{B.}$ $f^{\prime \prime}(0) < 0, g^{\prime \prime}(0) < 0$
$\text{C.}$ $f^{\prime \prime}(0)>0, g^{\prime \prime}(0)>0$
$\text{D.}$ $f^{\prime \prime}(0)>0, g^{\prime \prime}(0) < 0$
设 $I=\int_0^{\frac{\pi}{4}} \ln \sin x \mathrm{~d} x , J=\int_0^{\frac{\pi}{4}} \ln \cot x \mathrm{~d} x$ ,$K=\int_0^{\frac{\pi}{4}} \ln \cos x \mathrm{~d} x$ ,则 $I, J, K$ 的大小关系是
$\text{A.}$ $I < J < K$
$\text{B.}$ $I < K < J$
$\text{C.}$ $J < I < K$
$\text{D.}$ $K < J < I$
已知 $f(x)$ 在 $x=0$ 处可导,且 $f(0)=0$ ,则 $\lim _{x \rightarrow 0} \frac{x^2 f(x)-2 f\left(x^3\right)}{x^3}=$
$\text{A.}$ $-2 f^{\prime}(0)$
$\text{B.}$ $-f^{\prime}(0)$
$\text{C.}$ $f^{\prime}(0)$
$\text{D.}$ $0$
设 $I=\int_0^{\frac{\pi}{4}} \ln \sin x \mathrm{~d} x , J=\int_0^{\frac{\pi}{4}} \ln \cot x \mathrm{~d} x$ ,$K=\int_0^{\frac{\pi}{4}} \ln \cos x \mathrm{~d} x$ ,则 $I, J, K$ 的大小关系是
$\text{A.}$ $I < J < K$
$\text{B.}$ $I < K < J$
$\text{C.}$ $J < I < K$
$\text{D.}$ $K < J < I$
设函数 $f(x)=\left(e^x-1\right)\left(e^{2 x}-2\right) \cdots\left(e^{n x}-n\right)$ ,其中 $n$为正整数,则 $f^{\prime}(0)=$
$\text{A.}$ $(-1)^{n-1}(n-1)$ !
$\text{B.}$ $(-1)^n(n-1)$ !
$\text{C.}$ $(-1)^{n-1} n$ !
$\text{D.}$ $(-1)^n n$ !
设 $I_k=\int_0^{k \pi} e^{x^2} \sin x \mathrm{~d} x(k=1,2,3)$ ,则有
$\text{A.}$ $I_1 < I_2 < I_3$
$\text{B.}$ $I_3 < I_2 < I_1$
$\text{C.}$ $I_2 < I_3 < I_1$
$\text{D.}$ $I_2 < I_1 < I_3$
设函数 $f(x)=\left(e^x-1\right)\left(e^{2 x}-2\right) \cdots\left(e^{n x}-n\right)$ ,其中 $n$为正整数,则 $f^{\prime}(0)=$
$\text{A.}$ $(-1)^{n-1}(n-1)$ !
$\text{B.}$ $(-1)^n(n-1)$ !
$\text{C.}$ $(-1)^{n-1} n$ !
$\text{D.}$ $(-1)^n n$ !
设 $I_k=\int_0^{k \pi} e^{x^2} \sin x \mathrm{~d} x(k=1,2,3)$ ,则有
$\text{A.}$ $I_1 < I_2 < I_3$
$\text{B.}$ $I_3 < I_2 < I_1$
$\text{C.}$ $I_2 < I_3 < I_1$
$\text{D.}$ $I_2 < I_1 < I_3$
设函数 $f(x)=\left(e^x-1\right)\left(e^{2 x}-2\right) \cdots\left(e^{n x}-n\right)$ ,其中 $n$为正整数,则 $f^{\prime}(0)=$
$\text{A.}$ $(-1)^{n-1}(n-1)$ !
$\text{B.}$ $(-1)^n(n-1)$ !
$\text{C.}$ $(-1)^{n-1} n$ !
$\text{D.}$ $(-1)^n n$ !
设函数 $f(x)=\left\{\begin{array}{l}\frac{1}{(x-1)^{\alpha-1}}, 1 < x < e \\ \frac{1}{x \ln ^{\alpha+1} x}, x \geq e\end{array}\right.$, 若反常积分 $\int_1^{+\infty} f(x) \mathrm{d} x$ 收敛,则
$\text{A.}$ $\alpha < -2$
$\text{B.}$ $\alpha>2$
$\text{C.}$ $-2 < \alpha < 0$
$\text{D.}$ $0 < \alpha < 2$
设函数 $f(x)$ 具有二阶导数, $g(x)=f(0)(1-x)+f(1) x$ ,则在 $[0,1]$ 上
$\text{A.}$ 当 $f^{\prime}(x) \geq 0$ 时, $f(x) \geq g(x)$
$\text{B.}$ 当 $f^{\prime}(x) \geq 0$ 时, $f(x) \leq g(x)$
$\text{C.}$ 当 $f^{\prime \prime}(x) \geq 0$ 时, $f(x) \geq g(x)$
$\text{D.}$ 当 $f^{\prime \prime}(x) \geq 0$ 时, $f(x) \leq g(x)$
若函数 $\int_{-\pi}^\pi\left(x-a_1 \cos x-b_1 \sin x\right)^2 \mathrm{~d} x$
$$
=\min _{a, b \in \mathrm{R}}\left\{\int_{-\pi}^\pi(x-a \cos x-b \sin x)^2 \mathrm{~d} x\right\} \text {, }
$$
则 $a_1 \cos x+b_1 \sin x=$
$\text{A.}$ $2 \sin x$
$\text{B.}$ $2 \cos x$
$\text{C.}$ $2 \pi \sin x$
$\text{D.}$ $2 \pi \cos x$
设函数 $f(x)$ 具有二阶导数, $g(x)=f(0)(1-x)+f(1) x$ ,则在 $[0,1]$ 上
$\text{A.}$ 当 $f^{\prime}(x) \geq 0$ 时, $f(x) \geq g(x)$
$\text{B.}$ 当 $f^{\prime}(x) \geq 0$ 时, $f(x) \leq g(x)$
$\text{C.}$ 当 $f^{\prime \prime}(x) \geq 0$ 时, $f(x) \geq g(x)$
$\text{D.}$ 当 $f^{\prime \prime}(x) \geq 0$ 时, $f(x) \leq g(x)$
曲线 $\left\{\begin{array}{l}x=t^2+7 \\ y=t^2+4 t+1\end{array}\right.$ 上对应于 $t=1$ 的点处的曲率半径是
$\text{A.}$ $\frac{\sqrt{10}}{50}$
$\text{B.}$ $\frac{\sqrt{10}}{100}$
$\text{C.}$ $10 \sqrt{10}$
$\text{D.}$ $5 \sqrt{10}$
设函数 $f(u, v)$ 满足 $f\left(x+y, \frac{y}{x}\right)=x^2-y^2$ ,则 $\left.\frac{\partial f}{\partial u}\right|_{\substack{u=1 \\ v=1}}$与 $\left.\frac{\partial f}{\partial v}\right|_{\substack{u=1 \\ v=1}}$ 依次是
$\text{A.}$ $\frac{1}{2}, 0$
$\text{B.}$ $0, \frac{1}{2}$
$\text{C.}$ $-\frac{1}{2}, 0$
$\text{D.}$ $0,-\frac{1}{2}$
若反常积分 $\int_0^{+\infty} \frac{1}{x^a(1+x)^b} \mathrm{~d} x$ 收敛,则
$\text{A.}$ $a < 1$ 且 $b>1$
$\text{B.}$ $a>1$ 且 $b>1$
$\text{C.}$ $a < 1$ 且 $a+b>1$
$\text{D.}$ $a>1$ 且 $a+b>1$
已知函数 $f(x)=\left\{\begin{array}{c}2(x-1), x < 1, \\ \ln x, \quad x \geq 1,\end{array}\right.$ 则 $f(x)$ 的一个原函数为
$\text{A.}$ $F(x)=\left\{\begin{array}{l}(x-1)^2, x < 1, \\ x(\ln x-1), x \geq 1\end{array}\right.$
$\text{B.}$ $F(x)=\left\{\begin{array}{l}(x-1)^2, x < 1, \\ x(\ln x+1)-1, x \geq 1\end{array}\right.$
$\text{C.}$ $F(x)=\left\{\begin{array}{l}(x-1)^2, x < 1 \\ x(\ln x+1)+1, x \geq 1\end{array}\right.$
$\text{D.}$ $F(x)=\left\{\begin{array}{l}(x-1)^2, x < 1, \\ x(\ln x-1)+1, x \geq 1\end{array}\right.$
已知函数 $f(x)=\left\{\begin{array}{cc}2(x-1), & x < 1, \\ \ln x, & x \geq 1,\end{array}\right.$ 则 $f(x)$ 的一个原函数为
$\text{A.}$ $F(x)=\left\{\begin{array}{l}(x-1)^2, x < 1 \\ x(\ln x-1), x \geq 1\end{array}\right.$
$\text{B.}$ $F(x)=\left\{\begin{array}{l}(x-1)^2, x < 1, \\ x(\ln x+1)-1, x \geq 1\end{array}\right.$
$\text{C.}$ $F(x)=\left\{\begin{array}{l}(x-1)^2, x < 1, \\ x(\ln x+1)+1, x \geq 1\end{array}\right.$
$\text{D.}$ $F(x)=\left\{\begin{array}{l}(x-1)^2, x < 1, \\ x(\ln x-1)+1, x \geq 1\end{array}\right.$
反常积分(1) $\int_{-\infty}^0 \frac{1}{x^2} e^{\frac{1}{x}} \mathrm{~d} x$, (2) $\int_0^{+\infty} \frac{1}{x^2} e^{\frac{1}{x}} \mathrm{~d} x$ 的敛散性为
$\text{A.}$ (1)收敛(2)收敛
$\text{B.}$ (1)收敛(2)发散
$\text{C.}$ (1)收敛(2)收敛
$\text{D.}$ (1)发散(2)发散
设函数 $f(x)$ 在 $(-\infty,+\infty)$ 内连续,其导函数的图形如图所示, 则

$\text{A.}$ 函数 $f(x)$ 有 2 个极值点,曲线 $y=f(x)$ 有 2 个拐点
$\text{B.}$ 函数 $f(x)$ 有 2 个极值点,曲线 $y=f(x)$ 有 3 个拐点
$\text{C.}$ 函数 $f(x)$ 有 3 个极值点,曲线 $y=f(x)$ 有 1 个拐点
$\text{D.}$ 函数 $f(x)$ 有 3 个极值点,曲线 $y=f(x)$ 有 2 个拐点
设函数 $f_i(x)(i=1,2)$ 具有二阶连续导数,且 $f_i^{\prime \prime}\left(x_0\right) < 0(i=1,2)$ ,若两条曲线 $y=f_i(x)(i=1,2)$ 在点 $\left(x_0, y_0\right)$ 处具有公切线 $y=g(x)$ ,且在该点处曲线 $y=f_1(x)$ 的曲率大于曲率 $y=f_2(x)$ 的曲率,则在 $x_0$ 的某个邻域内,有
$\text{A.}$ $f_1(x) \leq f_2(x) \leq g(x)$
$\text{B.}$ $f_2(x) \leq f_1(x) \leq g(x)$
$\text{C.}$ $f_1(x) \leq g(x) \leq f_2(x)$
$\text{D.}$ $f_2(x) \leq g(x) \leq f_1(x)$
已知函数 $f(x, y)=\frac{e^x}{x-y}$ ,则
$\text{A.}$ $f_x{ }^{\prime}-f_y^{\prime}=0$
$\text{B.}$ $f_x^{\prime}+f_y^{\prime}=0$
$\text{C.}$ $f_x^{\prime}-f_y^{\prime}=f$
$\text{D.}$ $f_x^{\prime}+f_y^{\prime}=f$
设 $J_i=\iint_{D_i} \sqrt[3]{x-y} \mathrm{~d} x \mathrm{~d} y(i=1,2,3)$ ,其中
$$
\begin{gathered}
D_1=\{(x, y) \mid 0 \leq x \leq 1,0 \leq y \leq 1\} \\
D_2=\{(x, y) \mid 0 \leq x \leq 1,0 \leq y \leq \sqrt{x}\}, \\
D_3=\left\{(x, y) \mid 0 \leq x \leq 1, x^2 \leq y \leq 1\right\},
\end{gathered}
$$
则
$\text{A.}$ $J_1 < J_2 < J_3$
$\text{B.}$ $J_3 < J_1 < J_2$
$\text{C.}$ $J_2 < J_3 < J_1$
$\text{D.}$ $J_2 < J_1 < J_3$
函数 $f(x, y, z)=x^2 y+z^2$ 在点 $(1,2,0)$ 处沿向量 $\vec{n}=(1,2,2)$ 的方向导数为
$\text{A.}$ 12
$\text{B.}$ 6
$\text{C.}$ 4
$\text{D.}$ 2
甲乙两人赛跑,计时开始时,甲在乙前方 10 (单位:m) 处.图中,实线表示甲的速度曲线 $v=v_1(t)$ (单位: $\mathrm{m} / \mathrm{s}$ )虚线表示乙的速度曲线 $v=v_2(t)$ ,三块阴影部分面积的数值依次为 $10 , 20 , 3$ ,计时开始后乙追上甲的时刻记为 $t_0$ (单位: $s$ ),则
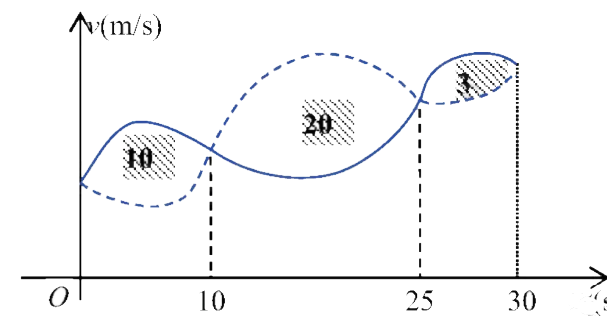
$\text{A.}$ $t_0=10$
$\text{B.}$ $15 < t_0 < 20$
$\text{C.}$ $t_0=25$
$\text{D.}$ $t_0>25$
设函数 $f(x)$ 可导,且 $f(x) f^{\prime}(x)>0$ ,则
$\text{A.}$ $f(1)>f(-1)$
$\text{B.}$ $f(1) < f(-1)$
$\text{C.}$ $|f(1)|>|f(-1)|$
$\text{D.}$ $|f(1)| < |f(-1)|$
过点 $(1,0,0)$ 与 $(0,1,0)$ 且与 $z=x^2+y^2$ 相切的平面方程为
$\text{A.}$ $z=0$ 与 $x+y-z=1$
$\text{B.}$ $z=0$ 与 $2 x+2 y-z=2$
$\text{C.}$ $y=x$ 与 $x+y-z=1$
$\text{D.}$ $y=x$ 与 $2 x+2 y-z=2$
设 $M=\int_{-\frac{\pi}{2}}^{\frac{\pi}{2}} \frac{(1+x)^2}{1+x^2} \mathrm{~d} x$ ,
$$
N=\int_{-\frac{\pi}{2}}^{\frac{\pi}{2}} \frac{1+x}{e^x} \mathrm{~d} x, K=\int_{-\frac{\pi}{2}}^{\frac{\pi}{2}}(1+\sqrt{\cos x}) \mathrm{d} x ,
$$
则 $M, N, K$ 的大小关系为
$\text{A.}$ $M>N>K$
$\text{B.}$ $M>K>N$
$\text{C.}$ $K>M>N$
$\text{D.}$ $K>N>M$
设 $M=\int_{-\frac{\pi}{2}}^{\frac{\pi}{2}} \frac{(1+x)^2}{1+x^2} \mathrm{~d} x$,
$$
N=\int_{-\frac{\pi}{2}}^{\frac{\pi}{2}} \frac{1+x}{e^x} \mathrm{~d} x, K=\int_{-\frac{\pi}{2}}^{\frac{\pi}{2}}(1+\sqrt{\cos x}) \mathrm{d} x ,
$$
则 $M, N, K$ 的大小关系为
$\text{A.}$ $M>N>K$
$\text{B.}$ $M>K>N$
$\text{C.}$ $K>M>N$
$\text{D.}$ $K>N>M$