一、单选题 (共 40 题 ),每题只有一个选项正确
1. 若 , 则
.
.
.
2. 函数 的可去间断点的个数为
1
2
3
4
3. 设对于任意 , 方程 有两个不同的实根, 则 的取值范围 是
.
.
.
.
4. 函数 是
在 单调增加函数
在 单调减少函数
在 单增 单减函数
在 单减 单增函数
5. 如果一个二元函数 可以写为一个关于 的函数 乘以一个关于 的函数 , 也就是 的形式, 我们把符合这样的情况的函数叫做 “二元函数 关于变量 可分离”, 假定下列的函数中 具有二阶连续偏导数, 则下列说法中不正确的是 ( )
(1). 若 , 则 关于变量 可分离
(2). 若 , 则 关于变量 可分离
(3). 若 并且 , 则 关于变量 可分离
(4.) 若 并且满足 , 则 关于变量 可分离
(2)
(1)(3)(4)
(2)(4)
(1)(3)
6. 下列有关定义在 上的可导函数 的说法正确的是
若 , 并且 , 使得 并且 , 使得 , 那么 在 内有最大值和最小值。
若 是奇函数, 并且 , 则 的斜渐近线条数一定是偶数。
若 并且 , 则
令 , 其中 , 则 存在
7. 设函数 且 , 则 在点 处
连续但不可导.
可导但 .
极限存在但不连续.
可微且 .
8. 设 在 的邻域内二阶连续可导, 且 , 则下列结论正确的是
为 的极小值点
为 的极大值点
为 的拐点
既不是 的极值点, 也不是 的拐点
9. 设连续函数 在 点可导, 且 , 若
则 在点 处
不连续, 是其第二类间断点.
不连续, 是其可去间断点.
连续,但不可导.
可导, 且 .
10. 设 则偶函数 有两个间断点 , 且
在 点左连续.
在 点右连续.
在 点左连续, 在 点右连续.
在 点右连续, 在 点左连续.
11. 设 , 则
12. 设连续函数 满足 , 则
2
3
4
-4
13. 设曲线
, 其中
为连续函数,
的图象如图所示, 则

有一个极大值点, 两个极小值点, 曲线 有两个拐点
有两个极大值点, 一个极小值点, 曲线 有两个拐点
有一个极大值点, 一个极小值点, 曲线 有两个拐点
有两个极大值点, 一个极小值点, 曲线 有一个拐点
14. 设 在 处连续, 且 , 则
是 的极小值点.
是 的极大值点.
是曲线 的拐点.
在 的邻域内单调.
15. 是函数 的
可去间断点
跳跃间断点
连续点
无穷间断点
16. 函数
的定义域为
, 导函数
在
内的图像如图所示, 则函数
在
内有极小值点
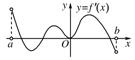
1个
2个
3个
4个
17. 设 在 处连续, 则 在 处可导的充分条件是
存在.
存在.
存在.
存在.
18. 函数 有
两个可去间断点
两个无穷间断点
一个可去间断点和一个跳跃间断点
一个可去间断点和一个无穷间断点
19. 设函数 在 上有定义, 且满足 , 则下列正确的是 ( )
.
.
.
.
20. 设 , 则
为 的第一类间断点.
为 的第一类间断点.
为 的第二类间断点.
为 的第二类间断点.
21. 已知函数 , 其中 在点 的某邻域内有定义, 则 在点 处偏导数存在的充分条件是
.
存在.
存在且 .
在点 处连续, 且 .
22. 设 , 则下列结论正确的是
仅有 2 个间断点, 为连续的偶函数.
仅有 2 个间断点, 为连续的奇函数.
有 3 个间断点, 有 3 个不可导点.
有 3 个间断点, 有 2 个不可导点.
23. 设 表示不超过 的最大整数,则 是函数 的
跳跃间断点
可去间断点
无穷型间断点
无限振荡型间断点
24. 函数 的间断点及类型是
是第一类间断点, 是第二类间断点
是第二类间断点, 是第一类间断点
均是第一类间断点
均是第二类间断点
25. 设函数 在 处连续, 下列命题错误 的是
若 存在, 则 .
若 存在, 则 .
若 存在, 则 存在.
若 存在, 则 存在.
26. 设函数
有两个极大值点, 无极小值点.
有一个极大值点, 一个极小值点.
有两个极大值点,一个极小值点.
有一个极大值点, 两个极小值点.
27. 设 是严格单调的连续奇函数, 是偶函数, 已知数列 , 则
当 存在时, 存在
当 存在时, 存在
当 存在时, 存在, 但 不一定存在
当 存在时, 存在, 但 不一定存在
28. 函数 的可去间断点的个数为
0
1
2
3
29. 设 为非零整数, 函数 在 上连续, 且 存在, 则
1
-1
2
-2
30.
31. 关于函数 给出以下结论:
(1) 当 时, 在点 处连续, 且偏导数存在;
(2) 当 时, 在点 处可微;
(3) 当 时, 在点 处连续;
(4) 当 时, 在点 处沿任意方向的方向导数均存在.
其中正确的个数为
4
3
2
1
32. 已知 , 则下列说法正确的是
有一个跳跃间断点,一个可去间断点和一个无穷间断点
且或 在闭区间 上有界
且或 在开区间 内不可积
记 , 则 在开区间 内可导
33. 设 表示不超过 的最大整数, 则 是函数 的
跳跃间断点
可去间断点
无穷型间断点
无限振荡型间断点
34. 设 在 上连续, 关于 的定积分有以下命题
(1) 若 且不恒等于 0 , 则
(2) 若 , 且 , 则
(3) 若 且存在 使 , 则
(4) 若 且 , 则 以上命题中正确的个数为 ( ).
1
2
3
4
35. 设函数 , 则
函数 有 3 个极值点, 曲线 有 4 个拐点.
函数 有 3 个极值点, 曲线 有 2 个拐点.
函数 有 1 个极值点, 曲线 有 2 个拐点.
函数 有 1 个极值点, 曲线 有 4 个拐点.
36. 函数 的不可导点的个数为
1
2
3
4
37. 已知 , 则
38. 设 是严格单调的连续奇函数, 是偶函数, 已知数列 , 则
当 存在时, 存在
当 存在时, 存在
当 存在时, 存在, 但 不一定存在
当 存在时, 存在, 但 不一定存在
39. 设 , 则当 时, 有
与 是等价无穷小
与 同阶但非等价无穷小
是比 高阶的无穷小
是比 低阶的无穷小
40. 设则 是 的
可去间断点
跳跃间断点
第二类间断点
连续点