一、单选题 （共 20 题，每小题 5 分，共 50 分，每题只有一个选项正确）
已知 $\cos (\alpha+\beta)=m, \tan \alpha \tan \beta=2$, 则 $\cos (\alpha-\beta)=(\quad)$
$\text{A.}$ $-3 m$
$\text{B.}$ $-\frac{m}{3}$
$\text{C.}$ $\frac{m}{3}$
$\text{D.}$ $3 m$
当 $x \in[0,2 \pi]$ 时, 曲线 $y=\sin x$ 与 $y=2 \sin \left(3 x-\frac{\pi}{6}\right)$ 的交点个数为
$\text{A.}$ 3
$\text{B.}$ 4
$\text{C.}$ 6
$\text{D.}$ 8
已知 $\cos \alpha \cos \beta=\frac{2}{5}, \cos (\alpha-\beta)=\frac{3}{5}$, 则 $\cos (\alpha+\beta)=$
$\text{A.}$ $\frac{1}{5}$
$\text{B.}$ $\frac{1}{3}$
$\text{C.}$ $\frac{4}{5}$
$\text{D.}$ $\frac{2}{3}$
在 $\triangle A B C$ 中, 角 $A, B, C$ 所对的边分别为 $a, b, c$, 若 $C=\frac{\pi}{3}, b+b \cos A=a \cos B$, 则 $B$ 的大小为
$\text{A.}$ $\frac{\pi}{6}$
$\text{B.}$ $\frac{\pi}{3}$
$\text{C.}$ $\frac{\pi}{9}$
$\text{D.}$ $\frac{2 \pi}{9}$
若 $\sin (\pi-\alpha)=\frac{4}{5}, \alpha$ 为第二象限角, 则 $\sin 2 \alpha=$
$\text{A.}$ $-\frac{7}{25}$
$\text{B.}$ $-\frac{24}{25}$
$\text{C.}$ $\frac{7}{25}$
$\text{D.}$ $\frac{24}{25}$
方程 $\sin 2 x=\sin x$ 在区间 $(0,2 \pi)$ 内的解的个数是
$\text{A.}$ 1
$\text{B.}$ 2
$\text{C.}$ 3
$\text{D.}$ 4
已知如图是函数 $\mathrm{y}=2 \sin \left(\omega_{\mathrm{x}}+\phi\right)\left(|\phi| < \frac{\pi}{2}\right)$ 的图象, 那么
$\text{A.}$ $\omega=\frac{10}{11}, \phi=\frac{\pi}{6} $
$\text{B.}$ $\omega=\frac{10}{11}, \phi=-\frac{\pi}{6} $
$\text{C.}$ $\omega=2, \phi=\frac{\pi}{6} $
$\text{D.}$ $\omega=2, \phi=-\frac{\pi}{6} $
函数 $y=\sin \left(2 x +\frac{5 \pi}{2}\right)$ 的图像的一条对称轴的方程是
$\text{A.}$ $x=-\frac{\pi}{2}$
$\text{B.}$ $x=-\frac{\pi}{4}$
$\text{C.}$ $x=\frac{\pi}{8}$
$\text{D.}$ $x=\frac{5 \pi}{4}$
如果函数 $\mathrm{y}=\sin \left(\omega_{\mathrm{x}}\right) \cos \left(\omega_{\mathrm{x}}\right)$ 的最小正周期是 $4 \pi$, 那么常数 $\omega$ 为
$\text{A.}$ 4
$\text{B.}$ 2
$\text{C.}$ $\frac{1}{2}$
$\text{D.}$ $\frac{1}{4}$
方程 $\sin 4 x \cos 5 x=-\cos 4 x \sin 5 x$ 的一个解是
$\text{A.}$ $10^{\circ}$
$\text{B.}$ $20^{\circ}$
$\text{C.}$ $50^{\circ}$
$\text{D.}$ $70^{\circ}$
函数 $\mathrm{f}({x})=\sin {x}+\cos {x}$ 的最小正周期是
$\text{A.}$ $2 \pi$
$\text{B.}$ $2 \sqrt{2} \pi$
$\text{C.}$ $\pi$
$\text{D.}$ $\frac{\pi}{4}$
$\sin 20^{\circ} \cos 70^{\circ}+\sin 10^{\circ} \sin 50^{\circ}$ 的值是
$\text{A.}$ $\frac{1}{4}$
$\text{B.}$ $\frac{\sqrt{3}}{2}$
$\text{C.}$ $\frac{1}{2}$
$\text{D.}$ $\frac{\sqrt{3}}{4}$
在下列函数中, 以 $\frac{\pi}{2}$ 为周期的函数是
$\text{A.}$ $y=\sin 2 x+\cos 4 x$
$\text{B.}$ $y=\sin 2 x \cos 4 x$
$\text{C.}$ $y=\sin 2 x+\cos 2 x$
$\text{D.}$ $y=\sin 2 x \cos 2 x$
当 $-\frac{\pi}{2} \leq x \leq \frac{\pi}{2}$ 时,函数 $f(x)=\sin x+\sqrt{3} \cos x$ 的
$\text{A.}$ 最大值是 1 , 最小值是 -1
$\text{B.}$ 最大值是 1 , 最小值是 $-\frac{1}{2}$
$\text{C.}$ 最大值是 2 , 最小值是 -2
$\text{D.}$ 最大值是 2 , 最小值是 -1
函数 $y=\operatorname{tg}\left(\frac{1}{2} x-\frac{1}{2} \pi\right)$ 在一个周期内的图像是
$\text{A.}$ 
$\text{B.}$ 
$\text{C.}$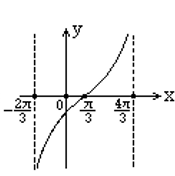 
$\text{D.}$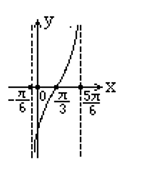 
函数 $y=\sin \left(\frac{\pi}{3}-2 x\right)+\cos 2 x$ 的最小正周期是
$\text{A.}$ $\frac{\pi}{2}$
$\text{B.}$ $\pi$
$\text{C.}$ $2 \pi$
$\text{D.}$ $4 \pi$
函数 ${f}({x})={M} \sin (\omega {x}+\varrho) \quad(\omega>0)$ 在区间 $[{a}, {b}]$ 上是增函数，且 ${f}({a})=-{M}, {f}({b})$ $={M}$ ，则函数 ${g}({x})={M} \cos (\omega {x}+\varrho)$ 在 $[{a}, {b}]$ 上
$\text{A.}$ 是增函数
$\text{B.}$ 是減函数
$\text{C.}$ 可以取得最大值 $M$
$\text{D.}$ 可以取得最小值$-M$
若 $f(x) \sin x$ 是周期为的奇函数，则 $f(x)$ 可以是
$\text{A.}$ $\sin x$
$\text{B.}$ $\cos x$
$\text{C.}$ $\sin 2 x$
$\text{D.}$ $\cos 2 x$
若 $\sin a>\operatorname{tg} a>\operatorname{ctg} a(-\pi / 2 < a < \pi / 2)$, 则 $a \in$
$\text{A.}$ $(-\pi / 2,-\pi / 4)$
$\text{B.}$ $(-\pi / 4,0)$
$\text{C.}$ $(0, \pi / 4)$
$\text{D.}$ $(\pi / 4, \pi / 2)$
在 $\triangle A B C$ 中, 已知 $a=a \cos B+b \cos A=1, \sin C=\frac{\sqrt{2}}{2}$, 则
$\text{A.}$ $b=1$
$\text{B.}$ $b=\sqrt{2}$
$\text{C.}$ $c=\sqrt{2}$
$\text{D.}$ $c=\sqrt{3}$
二、多选题 （共 1 题，每小题 5 分，共 20 分, 每题有多个选项符合要求，全部选对得 5 分，部分选对得 2 分，有选错得 0 分）
对于函数 $f(x)=\sin 2 x$ 和 $g(x)=\sin \left(2 x-\frac{\pi}{4}\right)$, 下列正确的有
$\text{A.}$ $f(x)$ 与 $g(x)$ 有相同的零点
$\text{B.}$ $f(x)$ 与 $g(x)$ 有相同的最大值
$\text{C.}$ $f(x)$ 与 $g(x)$ 有相同的最小正周期
$\text{D.}$ $f(x)$ 与 $g(x)$ 的图像有相同的对称轴
三、填空题 （共 9 题, 每小题 5 分，共 20 分， 请把答案直接填写在答题纸上）
已知 $\alpha$ 为第一象限角, $\beta$ 为第三像限角, $\tan \alpha+\tan \beta=4, \tan \alpha \tan \beta=\sqrt{2}+1$, 则 $\sin (\alpha+\beta)=$
设 $\triangle A B C$ 的内角 $A, B, C$ 所对边的长分别是 $a, b, c$, 且 $A=2 B, b \neq c, D$ 为 $B C$ 边上的中点, 且 $A D=\sqrt{2} \mathrm{c}$, 则 $\cos \mathrm{A}=$
将函数 $f(x)=\sin \left(2 x-\frac{\pi}{3}\right)$ 的图象沿 $x$ 轴向左平移 $\frac{\pi}{6}$ 个单位长度后得到函数 $g(x)$ 的图象, 则 $g\left(\frac{\pi}{4}\right)$ 的值为
$\triangle A B C$ 为锐角三角形, 其三个内角 $A 、 B、 C$ 的对边分别为 $a, b ,c$, 且 $b=1, C=2 B$, 则 $\triangle A B C$ 周长的取值范围为
$\sin 15^{\circ} \sin 75^{\circ}$ 的值是
已知 $\sin \theta+\cos \theta=\frac{1}{5}, \theta \in(0, \pi)$, 则 $\operatorname{ctg} \theta$ 的值是
$\operatorname{tg} 20^{\circ}+\operatorname{tg} 40^{\circ}+\sqrt{3} \operatorname{tg} 20^{\circ} \operatorname{tg} 40^{\circ}$ 的值是
$\frac{\sin 7^{\circ}+\cos 15^{\circ} \sin 8^{\circ}}{\cos 7^{\circ}-\sin 15^{\circ} \sin 8^{\circ}}$ 的值为
关于函数 $f(x)=4 \sin \left(2 x+\frac{\pi}{3}\right)(x \in R)$ ，有下列命题：
(1) 由 $f\left(x_1\right)=f\left(x_2\right)=0$ 可得 $x_1-x_2$ 必是 $\pi$ 的整数倍;
(2) $y=f(x)$ 的表达式可改写为 $y=4 \cos \left(2 x-\frac{\pi}{6}\right)$ ；
(3) $y=f(x)$ 的图象关于点 $\left(-\frac{\pi}{6}, 0\right)$ 对称;
(4) $y=f(x)$ 的图象关于直线 $x=-\frac{\pi}{6}$ 对称。
其中正确的命题的序号是_。(注: 把你认为正确的命题的序号都填上。)
四、解答题 （ 共 9 题，满分 80 分，解答过程应写出必要的文字说明、证明过程或演算步骤 ）
记 $\triangle A B C$ 的内角 $A, B, C$ 的对边分别为 $a, b, c$, 已知 $\sin C=\sqrt{2} \cos B, a^2+b^2-c^2=\sqrt{2} a b$
（1）求 $B$;
（2）若 $\triangle A B C$ 的面积 $3+\sqrt{3}$, 求 $c$
记 $\triangle A B C$ 的内角 $A, B, C$ 的对边分别为 $a, b, c$, 已知 $\sin A+\sqrt{3} \cos A=2$.
(1) 求 $A$.
(2) 若 $a=2, \sqrt{2} b \sin C=c \sin 2 B$, 求 $\triangle A B C$ 的周长.
已知 $\sin a+\sin \beta=\frac{1}{4}, \cos a+\cos \beta=\frac{1}{3}$, 求 $ \tan(a+\beta)$ 的值.
已知 $\frac{\pi}{2} < \beta < \alpha < \frac{3 \pi}{4}, \cos (\alpha-\beta)=\frac{12}{13}, \sin (\alpha+\beta)=-\frac{3}{5}$. 求 $\sin 2 a$ 的值.
已知函数 $f(x)=\operatorname{tg} x, x \in\left(0, \frac{\pi}{2}\right)$. 若 $x_1, x_2 \in\left(0, \frac{\pi}{2}\right)$, 且 $x_1 \neq x_2$, 证明 $\frac{1}{2}\left[f\left(x_1\right)+f\left(x_2\right)\right]>f\left(\frac{x_1+x_2}{2}\right)$
求函数 $y=\frac{\sin 3 x \sin ^3 x+\cos 3 x \cos ^3 x}{\cos ^2 2 x}+\sin 2 x$ 的最小值.
求 $\sin ^2 20^{\circ}+\cos ^2 50^{\circ}+\sin 20^{\circ} \cos 50^{\circ}$ 的值.
已知 $\triangle A B C$ 的三个内角 $A, B, C$ 满足: $A+C=2 B, \frac{1}{\cos A}+\frac{1}{\cos C}=-\frac{\sqrt{2}}{\cos B}$, 求 $\cos \frac{A-C}{2}$ 的值.
在 $\triangle \mathrm{ABC}$ 中, $a, b, c$ 分别是角 $A, B, a$ 的对边, 设 $a+c=2 b, A-C=\frac{\pi}{3}$ ,求$\sin B$