单选题 (共 5 题 ),每题只有一个选项正确
设 $\lim _{x \rightarrow 0} \frac{\ln (1+x)-\left(a x+b x^2\right)}{x^2}=2$ ,则
$\text{A.}$ $a=1, b=-\frac{5}{2}$
$\text{B.}$ $a=0, b=-2$
$\text{C.}$ $a=0, b=-\frac{5}{2}$
$\text{D.}$ $a=1, b=-2$
设 $f(x)=\left\{\begin{array}{ll}\frac{2}{3} x^3, & x \leq 1 \\ x^2, & x>1\end{array}\right.$ ,则 $f(x)$ 在 $x=1$ 处的
$\text{A.}$ 左、右导数都存在
$\text{B.}$ 左导数存在,但右导数不存在
$\text{C.}$ 左导数不存在,但右导数存在
$\text{D.}$ 左、右导数都不存在
设 $y=f(x)$ 是满足微分方程 $y^{\prime \prime}+y^{\prime}-e^{\sin x}=0$ 的解,且 $f^{\prime}\left(x_0\right)=0$ ,则 $f(x)$ 在
$\text{A.}$ $x_0$ 的某个邻域内单调增加
$\text{B.}$ $x_0$ 某个邻域内单调减少
$\text{C.}$ $x_0$ 处取得极小值
$\text{D.}$ $x_0$ 处取得极大值
曲线 $y=e^{\frac{1}{x^2}} \arctan \frac{x^2+x-1}{(x-1)(x+2)}$ 的渐近线有
$\text{A.}$ 1
$\text{B.}$ 2
$\text{C.}$ 3
$\text{D.}$ 4
设 $M=\int_{-\frac{\pi}{2}}^{\frac{\pi}{2}} \frac{\sin x}{1+x^2} \cos ^4 x \mathrm{~d} x$ ,
$$
\begin{aligned}
& N=\int_{-\frac{\pi}{2}}^{\frac{\pi}{2}}\left(\sin ^3 x+\cos ^4 x\right) \mathrm{d} x, \\
& P=\int_{-\frac{\pi}{2}}^{\frac{\pi}{2}}\left(x^2 \sin ^3 x-\cos ^4 x\right) \mathrm{d} x
\end{aligned}
$$
则
$\text{A.}$ $N < P < M$
$\text{B.}$ $M < P < N$
$\text{C.}$ $N < M < P$
$\text{D.}$ $P < M < N$
填空题 (共 5 题 ),请把答案直接填写在答题纸上
若 $f(x)=\left\{\begin{array}{cl}\frac{\sin 2 x+e^{2 a x}-1}{x} & x \neq 0 \\ a & x=0\end{array}\right.$ 在 $(-\infty,+\infty)$ 上连续,则 $a=$
设函数 $y=y(x)$ 由参数方程 $\left\{\begin{array}{l}x=t-\ln (1+t) \\ y=t^3+t^2\end{array}\right.$ 所确定,则 $\frac{\mathrm{d}^2 y}{\mathrm{~d} x^2}=$
$\frac{\mathrm{d}}{\mathrm{d} x}\left[\int_0^{\cos 3 x} f(t) \mathrm{d} t\right]=$
$\int x^3 e^{x^2} \mathrm{~d} x=$
微分方程 $y \mathrm{~d} x+\left(x^2-4 x\right) \mathrm{d} y=0$ 的通解为
解答题 (共 10 题 ),解答过程应写出必要的文字说明、证明过程或演算步骤
设 $y=f(x+y)$ ,其中 $f$ 具有二阶导数,且其一阶导数不等于 1 ,求 $\frac{\mathrm{d}^2 y}{\mathrm{~d} x^2}$
计算 $\int_0^1 x\left(1-x^4\right)^{\frac{3}{2}} \mathrm{~d} x$.
计算 $\lim _{n \rightarrow \infty} \tan ^n\left(\frac{\pi}{4}+\frac{2}{n}\right)$.
求 $\int \frac{d x}{\sin (2 x)+2 \sin x}$.
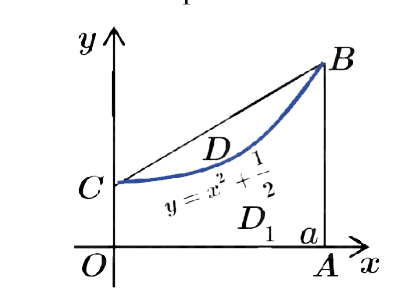
如图,设曲线方程为 $y=x^2+\frac{1}{2}$ ,梯形 $O A B C$ 的面积为 $D$ ,曲边梯形 $O A B C$ 的面积为 $D_1$ ,点 $A$ 的坐标为 $(a, 0), a>0$ ,证明: $\frac{D}{D_1} < \frac{3}{2}$.
设当 $x>0$ 时,方程 $k x+\frac{1}{x}=1$ 有且仅有一个解,求 $k$的取值范围.
设 $y=\frac{x^3+4}{x^2}$.
(1)求函数的增减区间及极值;
(2)函数图像的凹凸区间及拐点;
(3)求其渐近线;
(4)作出其图形.
求微分方程 $y^{\prime \prime}+a^2 y=\sin x$ 的通解,其中常数 $a>0$.
设 $f(x)$ 在 $[0,1]$ 上连续且递减,证明:当 $0 < \lambda < 1$ 时,
$$
\int_0^\lambda f(x) \mathrm{d} x \geq \lambda \int_0^1 f(x) \mathrm{d} x .
$$
求曲线 $y=3-\left|x^2-1\right|$ 与 $x$ 轴围成的封闭图形绕直线 $y=3$ 旋转所得的旋转体体积.