一、单选题 (共 35 题 ),每题只有一个选项正确
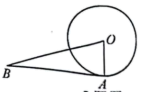
1. 已知平面内有 e 和点 , 若e 半径为 , 线段 , 则直线 与 e 的位置关系为 ( )
相离
相交
相切
相交或相切
2. 如图, 四边形
内接于
为
中点,
, 则
等于 ( )

3. 如图,
是
上一点,
是直径,
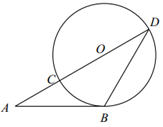
, 点
在
上且平分
, 则
的 长为 ( )

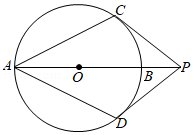
4. 如图,
为
的直径, 点
在
的延长线上,
与
相切, 切点分别为
. 若
, 则
等于( )
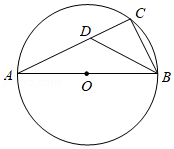
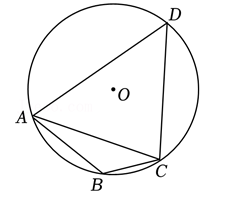
5. 如图, 点
在圆
上,
, 则

6. 如图,
是
的直径, 点
为圆上一点,
的平 分线交
于点
, 则
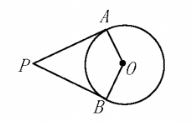
的直径为
1
2
7. 如图,
是
的切线,
、 为切点, 若
, 则
的度数为 ( )
8. 水中涟渏 (圆形水波) 不断扩大, 记它的半径为 , 则圆周长 与 的关系式为 . 下列判断正确的是()
2 是变量
是变量
是变量
是常量
9. 有一个正 边形旋转 后与自身重合, 则 为 ( )
6
9
12
15
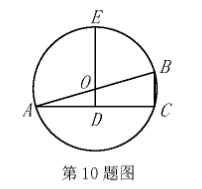
10. 如图,
的半径
为
于点
, 则
的长是 ( )
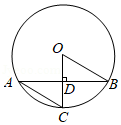
2
3
11. 如图,
是
的直径,
, 则
的半径为
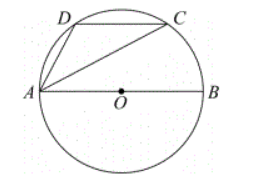
12. 如图, 四边形
中.
交
于点
, 以点
为圆心,
为 半径, 且
的圆交
于点
, 则阴影部分的面积为
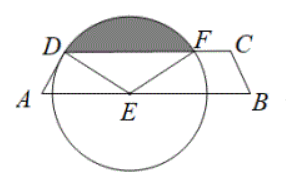
13. 如图, 四边形
是
的内接四边形,
是
的直径, 连 接
. 若
, 则
的度数是()

14. 如图, 是圆 的直径, 、、 都是圆上的点, 其中 、 在 下方, 在 上方, 则 等于
15. 如图, 在平面直角坐标系中, 边长为 2 的正六边形 ABCDEF 的中 心与原点
重合,
轴, 交
轴于点
, 将
绕点
顺时针旋转, 每次旋转
, 则第 2022 次旋转结束时, 点
的 坐标为
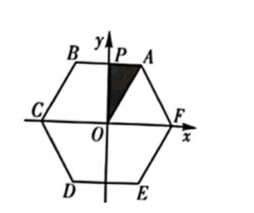
16. 如图,
是 圆
的切线,
为切点, 连接
交圆
于点
, 延长
交圆
于点
, 连接
. 若
, 且
, 则
的长度是
3
4
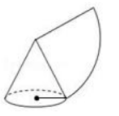
17. 如图, 圆锥的底面半径为 5 , 高为 12 , 则该圆锥的侧面积为
18. 如图,
是
的直径, 弦
交
于点
, 且
, 则
的长为
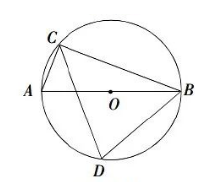
19. 如图,
、 是
上直径
两侧的两点, 若
, 则
的度数是
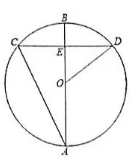
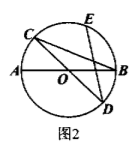
20. 如图 2,
是
的两条直径,
是劣弧
的中点, 连接
,
. 若
, 则
的度数为
21. 如图,
是
的直径,
垂直于弦
于点
的延长线交
于点
. 若
, 则
的长是
1
2
4
22. 如图,
是
的弦,
, 垂足为
,
, 则
的度数为
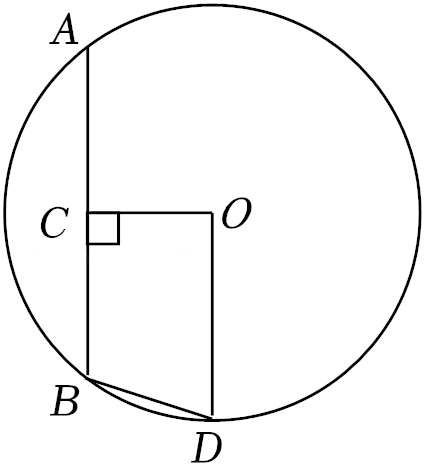
23. 如图, 已知
是
的直径,
是
的弦,
. 重足为
. 著
,则

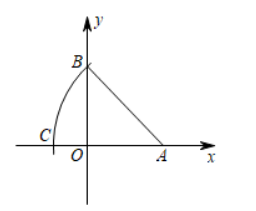
24. 如图所示,
, ,以点
为圆心,
长为半径画弧交
轴负半轴于点
,则点
的坐标为
25. 如图, 半径为 5 的 与正五边形 相切于点 、, 则弧 的长为
26. 已知线段 经过 、 两点, 若 , 则称点 是线段 的 “好心”; 上的 点称作线段 的 “闪光点”. 已知 .
(1)点 是线段 的 “好心”;
(2)若反比例函数 上存在线段 的 “好心”, 则 ;
(3)线段 的 “闪光点” 组成的图形既是轴对称图形, 又是中心对称图形;
(4)若直线 上存在线段 的 “闪光点”, 则 .
下来说法正确的是
(1)(2)(3)(4)
(1)(3)(4)
(1)(3)
(1)(2)
27. 图, 是 的切线, 为切点, 连接 交 于点 , 戛长 交 于点 , 连接 . 若 , 且 , 则 的长度是
3
4
28. 如图,
是
的切线,
切点, 连接
, 若
, 则
的度数为
29. 如图,
是半圆
的直径,
是半圆上的两点, 若
. 则
的大小为

30. 圆的直径是 14 , 若圆心与直线上某一点的距离是 7 , 则该直线和圆的位置关系是
相离
相切
相交
相交或相切
31. 如图, 四边形
内接于
, 则
的半径为
32. 如图,
内接于
是
的直径, 若
, 则
的度数是

33. 如图, 扇形纸片 的半径为 3 , 沿 折叠扇形纸片, 点 恰好落在 上的点 处, 图中阴影部分的面积为
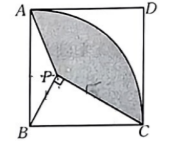
34. 如图. 四边形
为正方形, 以
为圆心,
长为半径画
为四边形内部一点,
, 连接
, 则阴影部分的面积为
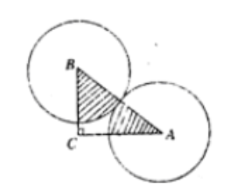
35. 中,
, 两等圆
、 外切, 那么图中两个扇形(即阴 影部分)的面积之和为
二、填空题 (共 5 题 ),请把答案直接填写在答题纸上
36. 一个扇形的圆心角是 , 半径为 4, 则这个扇形的面积为 ( ) . (结果保留 )
37. 若一个扇形的圆心角为 , 面积为 , 则这个扇形的弧长为 ( ) (结果保留 ).
38. 如图, 圆
的半径为
内接于圆
. 若
, 则
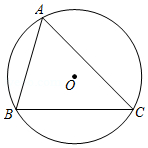
39. 如图,
是
的切线,
是切点. 若
, 则
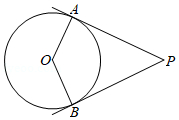
40. 如图, 从一块直径为
的圆形铁皮上剪出一圆心角为
的扇形, 则此扇形的面积为