一、单选题 (共 46 题,每小题 5 分,共 50 分,每题只有一个选项正确)
记 $S_{n}$ 为等差数列$ \{a_{n}\} $ 的前$n$项和. 已知 $ S_{4}=0, a_{5}=5 $ 则 ( )
$\text{A.}$ $a_n=2n-5$
$\text{B.}$ $a_n=3n-10$
$\text{C.}$ $S_n=2n^2-8n$
$\text{D.}$ $S_n=\dfrac{1}{2}n^2-2n$
已知等差数列 $\left\{a_{n}\right\}$ 满足 $a_{2}+a_{4}=4, a_{3}+a_{5}=10$, 则它的前 10 项的和 $S_{10}=$ ( )
$\text{A.}$ 138
$\text{B.}$ 135
$\text{C.}$ 95
$\text{D.}$ 23
已知 $\left\{a_{n}\right\}$ 为等比数列, $a_{4}+a_{7}=2, a_{5} a_{6}=-8$, 则 $a_{1}+a_{10}=$ ( )
$\text{A.}$ 7
$\text{B.}$ 5
$\text{C.}$ $-5$
$\text{D.}$ $-7$
设等差数列 $\left\{a_{n}\right\}$ 的前 $n$ 项和为 $S_{n}$, 若 $S_{m-1}=-2, S_{m}=0, S_{m+1}=3$, 则 $m=$ ( )
$\text{A.}$ 3
$\text{B.}$ 4
$\text{C.}$ 5
$\text{D.}$ 6
设 $\triangle A_{n} B_{n} C_{n}$ 的三边长分别为 $a_{n}, b_{n}, c_{n}, \triangle A_{n} B_{n} C_{n}$ 的面积为 $S_{n}, n=1$ , 2, 3...若 $b_{1}>c_{1}, b_{1}+c_{1}=2 a_{1}, a_{n+1}=a_{n}, b_{n+1}=\frac{c_{n}+a_{n}}{2}, c_{n+1}=\frac{b_{n}+a_{n}}{2}$, 则 ( )
$\text{A.}$ $\left\{\mathrm{S}_{\mathrm{n}}\right\}$ 为递减数列
$\text{B.}$ $\left\{\mathrm{S}_{\mathrm{n}}\right\}$ 为递增数列
$\text{C.}$ $\left\{\mathbf{S}_{2 n-1}\right\}$ 为递增数列, $\left\{\mathbf{S}_{2 n}\right\}$ 为递减数列
$\text{D.}$ $\left\{\mathrm{S}_{2 \mathrm{n}-1}\right\}$ 为递减数列, $\left\{\mathrm{S}_{2 \mathrm{n}}\right\}$ 为递增数列
记 $\mathrm{S}_{n}$ 为等差数列 $\left\{a_{n}\right\}$ 的前 $n$ 项和. 若 $a_{4}+a_{5}=24, S_{6}=48$, 则 $\left\{a_{n}\right\}$ 的公 差为 ( )
$\text{A.}$ 1
$\text{B.}$ 2
$\text{C.}$ 4
$\text{D.}$ 8
几位大学生响应国家的创业号召, 开发了一款应用软件. 为激发大 家学习数学的兴趣, 他们推出了 “解数学题获取软件激活码”的活动. 这款软 件的激活码为下面数学问题的答案:已知数列 1, 1, 2, 1,2,4,1,2,4, $8,1,2,4,8,16, \ldots$, 其中第一项是 $2^{0}$, 接下来的两项是 $2^{0}, 2^{1}$, 再接下来的三项是 $2^{0}, 2^{1}, 2^{2}$, 依此类推. 求满足如下条件的最小整数 $N: N>100$ 且该数列的前 $N$ 项和为 2 的整数幂. 那么该款软件的激活码是( )
$\text{A.}$ 440
$\text{B.}$ 330
$\text{C.}$ 220
$\text{D.}$ 110
记 $S_{n}$ 为等差数列 $\left\{a_{n}\right\}$ 的前 $n$ 项和. 若 $3 S_{3}=S_{2}+S_{4}, a_{1}=2$, 则 $a_{5}=$
$\text{A.}$ $-12$
$\text{B.}$ $-10$
$\text{C.}$ 10
$\text{D.}$ 12
在数列 $\left\{a_{n}\right\}$ 中, $a_{1}=2, a_{n+1}=a_{n}+\ln \left(1+\frac{1}{n}\right)$, 则 $a_{n}=$
$\text{A.}$ $2+\ln n$
$\text{B.}$ $2+(n-1) \ln n$
$\text{C.}$ $2+n \ln n$
$\text{D.}$ $1+n+\ln n$
在等差数列 $\left\{a_{n}\right\}$ 中, $a_{1}=1, a_{8}+a_{10}=10$, 则 $a_{5}=($ )
$\text{A.}$ 5
$\text{B.}$ 4
$\text{C.}$ 3
$\text{D.}$ 2
设 $a, b \in \mathbf{R}$, 数列 $\left\{a_{n}\right\}$ 中 $a_{n}=a, a_{n+1}=a_{n}^{2}+b, b \in \mathbf{N}^{*}$, 则
$\text{A.}$ 当 $b=, a_{10}>10$
$\text{B.}$ 当 $b=, a_{10}>10$
$\text{C.}$ 当 $b=-2, a_{10}>10$
$\text{D.}$ 当 $b=-4, a_{10}>10$
已知等差数列 $\left\{a_{n}\right\}$ 的通项公式为 $a_{n}=3-2 n$, 则它的公差为
$\text{A.}$ $-2$
$\text{B.}$ $-3$
$\text{C.}$ $2$
$\text{D.}$ $3$
设 $S_{n}$ 是等差数列 $\left\{a_{n}\right\}$ 的前 $n$ 项和, 已知 $a_{2}=3, a_{6}=11$, 则 $S_{7}$ 等于
$\text{A.}$ 13
$\text{B.}$ 35
$\text{C.}$ 49
$\text{D.}$ 63
在各项都为正数的等比数列 $\left\{a_{n}\right\}$ 中, 首项 $a_{1}=3$, 前三项和为 21 , 则 $a_{3}+a_{4}+a_{5}=$
$\text{A.}$ 33
$\text{B.}$ 72
$\text{C.}$ 84
$\text{D.}$ 189
若数列 $\left\{a_{n}\right\}$ 满足: $a_{n+1}=1-\frac{1}{a_{n}}$ 且 $a_{1}=2$, 则 $a_{2010}=$
$\text{A.}$ $-1$
$\text{B.}$ $1$
$\text{C.}$ $2$
$\text{D.}$ $\frac{1}{2}$
等差数列 $\left\{a_{n}\right\}$ 和 $\left\{b_{n}\right\}$ 的前 $n$ 项和分别为 $S_{n}$ 与 $T_{n}$, 对一切自然数 $n$, 都 有 $\frac{S_{n}}{T_{n}}=\frac{2 n}{3 n+1}$, 则 $\frac{a_{5}}{b_{5}}$ 等于
$\text{A.}$ $\frac{2}{3}$
$\text{B.}$ $\frac{9}{14}$
$\text{C.}$ $\frac{20}{31}$
$\text{D.}$ $\frac{11}{17}$
已知数列 $\left\{a_n\right\}$ 的前 $n$ 项和为 $S_n$, 满足 $a_1=1, a_2=3,2 \sqrt{S_n}=\sqrt{S_{n+1}}+\sqrt{S_{n-1}}(n \geq 2)$,
则 $a_{2022}=$
$\text{A.}$ 4043
$\text{B.}$ 4042
$\text{C.}$ 4041
$\text{D.}$ 4040
已知等比数列 $\left\{a_n\right\}$ 的各项均为正数, 且 $a_1 a_2 a_3=27, a_4-a_2=-\frac{8}{3}$, 则 $a_1 a_2 \cdots a_n$ 的最大值为
$\text{A.}$ 9
$\text{B.}$ 8
$\text{C.}$ 3
$\text{D.}$ 27
已知等差数列 $\left\{a_n\right\}$ 的前 $n$ 项和为 $S_n$, 且 $a_3+a_5=-10, S_6=-42$, 则 $S_{10}=$
$\text{A.}$ 6
$\text{B.}$ 10
$\text{C.}$ 12
$\text{D.}$ 20
已知 $\left\{a_n\right\}$ 为等差数列, $S_n$ 为其前 $n$ 项和, 则下列结论一定成立的是
$\text{A.}$ 若 $a_1=a_5$, 则 $a_1=a_2=\cdots=a_n$
$\text{B.}$ 若 $a_5>a_3$, 则 $S_1 < S_2 < \cdots < S_n$
$\text{C.}$ 若 $a_3=2$, 则 $a_1^2+a_5^2 \geqslant 8$
$\text{D.}$ 若 $a_4=8, a_8=4$, 则 $S_{12}=66$
已知等比数列 $\left\{a_n\right\}$ 的前 $n$ 项和为 $S_n$, 若 $S_3=4, S_6=12$, 则 $S_{12}=$
$\text{A.}$ 32
$\text{B.}$ 28
$\text{C.}$ 48
$\text{D.}$ 60
已知数列 $\left\{a_n\right\}$ 满足 $a_n \cdot(-1)^n+a_{n+2}=2 n-1, S_{20}=650$, 则 $a_{23}=$
$\text{A.}$ 231
$\text{B.}$ 234
$\text{C.}$ 279
$\text{D.}$ 276
设等比数列 $\left\{a_n\right\}$ 满足 $a_1+a_3=20, a_2+a_4=10$, 则使 $a_1 \cdot a_2 \cdot a_3 \cdots a_n$ 最大的 $n$ 为
$\text{A.}$ 4
$\text{B.}$ 5
$\text{C.}$ 4 或 5
$\text{D.}$ 6
已知数列 $\left\{a_n\right\},\left\{b_n\right\}$ 均为公差不为 0 的等差数列, 且满足 $a_3=b_2, a_6=b_4$, 则 $\frac{a_4-a_1}{b_3-b_2}=( )$
$\text{A.}$ 2
$\text{B.}$ 1
$\text{C.}$ $\frac{3}{2}$
$\text{D.}$ 3
记 $S_n$ 为各项均为正数的等比数列 $\left\{a_n\right\}$ 的前 $n$ 项和, $S_3=\frac{7}{8}, a_3=\frac{1}{2}$, 则 $a_5=$ ( )
$\text{A.}$ $\frac{1}{4}$
$\text{B.}$ $\frac{1}{8}$
$\text{C.}$ 1
$\text{D.}$ 2
《天才引导的过程一一数学中的伟大定理》的作者威廉 - 邓纳姆曾写道: “如果你想要做 加法你需要 0 , 如果你想要做乘法你需要 1 , 如果你想要做微积分你需要 $\mathrm{e}$, 如果你想要做 几何你需要 $\pi$, 如果你想要做复分析你需要 $\mathrm{i}$, 这是数学的梦之队, 他们都在这个方程 里.” 这里指的方程就是: $\mathrm{e}^{x+\mathrm{i} y}=\mathrm{e}^x(\cos y+\mathrm{i} \sin y)$, 令 $x=0, y=\pi$, 则 $\mathrm{e}^{\mathrm{i} \pi}=-1$, 令 $x=0, y=n \pi$, 则 $\mathrm{e}^{\mathrm{i} n \pi}=\cos n \pi+\mathrm{i} \sin n \pi$, 若数列 $\left\{a_n\right\}$ 满足 $a_n=\mathrm{e}^{\mathrm{i} n \pi}, S_n$ 为数列 $\left\{a_n\right\}$ 的前 $n$ 项和, 则下列结论正确的个数是()
① $\left\{a_n\right\}$ 是等比数列
②$a_{2 n}=a_n^2$
③ $S_{21}=1$
④ $a_{n+2}=a_n$
$\text{A.}$ 1个
$\text{B.}$ 2个
$\text{C.}$ 3个
$\text{D.}$ 4个
在公比为正数的等比数列 $\left\{a_n\right\}$ 中, $a_2+a_4=30 ,a_4+a_6=\frac{15}{2}$, 则 $a_1$ 的值为
$\text{A.}$ 48
$\text{B.}$ 56
$\text{C.}$ 64
$\text{D.}$ 72
等差数列$a_n$的各项均为正数,首项与公差相等, $\sum_{k=1}^{15} \dfrac{1}{\sqrt{a_k}+\sqrt{n_{k+1}}}=2$, 则 $a_4$的值
$\text{A.}$ 6
$\text{B.}$ 7
$\text{C.}$ 8
$\text{D.}$ 9
在各项均为正整数,且满足下列条件的数量$a_n$中,$a_9$可能的最大值和最小值分别是$M$和$m$, 则$M+m$的值为
① $a_7=40$
② 对于任意正整数$n$,
$$
a_{n+2}= \begin{cases}a_{n+1}+a_n & \left(a_{n+1} \text { 不是3的倍数 }\right) \\ \frac{1}{3} a_{n+1} & \left(a_{n+1} \text { 是3的倍数 }\right)\end{cases}
$$
$\text{A.}$ 216
$\text{B.}$ 218
$\text{C.}$ 220
$\text{D.}$ 222
已知等差数列 $\left\{a_n\right\}$ 的前 $n$ 项和为 $S_n$, 且 $a_n>0$, 则 $\frac{S_6-S_3}{a_2+a_8}=$
$\text{A.}$ 2
$\text{B.}$ $\frac{3}{2}$
$\text{C.}$ 1
$\text{D.}$ $\frac{1}{2}$
已知实数 $a>0, b < 0$, 则 $\frac{\sqrt{3} b-a}{\sqrt{a^2+b^2}}$ 的取值范围是
$\text{A.}$ $[-2,-1)$
$\text{B.}$ $(-2,-1)$
$\text{C.}$ $(-2 .-1]$
$\text{D.}$ $[-2,-1]$
已知函数 $f(x)=\ln \frac{m+x}{1-n-x}(m>0, n>0)$ 是奇函数, 则 $\frac{1}{m}+\frac{2}{n}$ 的最小值为
$\text{A.}$ 3
$\text{B.}$ 5
$\text{C.}$ $3+2 \sqrt{2}$
$\text{D.}$ $3+4 \sqrt{2}$
若集合 $\left\{y \mid y=x+t\left(x^2-x\right), 0 \leq t \leq 1,1 \leq x \leq 2\right\}$ 表示的图形中, 两点间最大距离为 $d$ 面积为 $S$,则
$\text{A.}$ $d=3, \quad S < 1$
$\text{B.}$ $d=3, S>1$
$\text{C.}$ $d=\sqrt{10}, \quad S < 1$
$\text{D.}$ $d=\sqrt{10}, \quad S>1$
二次函数 $y=x^2+(a-1) x+1(a>0)$ 只有一个零点, 则不等式 $a x^2-8 x-a \geq 0$ 的解集为
$\text{A.}$ $\left\{x \left\lvert\,-\frac{1}{3} < x < 3\right.\right\}$
$\text{B.}$ $\left\{x \left\lvert\,-\frac{1}{3} \leq x \leq 3\right.\right\}$
$\text{C.}$ $\left\{x \left\lvert\, x < -\frac{1}{3}\right.\right.$ 或 $\left.x>3\right\}$
$\text{D.}$ $\left\{x \left\lvert\, x \leq-\frac{1}{3}\right.\right.$ 或 $\left.x \geq 3\right\}$
若关于 $x$ 的不等式 $x^2-4 x-a>0$ 在区间 $(1,5)$ 内有解, 则实数 $a$ 的取值范围是
$\text{A.}$ $(-\infty, 5)$
$\text{B.}$ $(5,+\infty)$
$\text{C.}$ $(-4,+\infty)$
$\text{D.}$ $(-\infty,-4)$
不等式 $a x^2-b x+c>0$ 的解集为 $\{x \mid-2 < x < 1\}$, 则函数 $y=a x^2+b x+c$ 的图像大致为
$\text{A.}$ 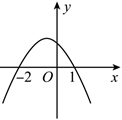 $\text{B.}$
$\text{B.}$  $\text{C.}$
$\text{C.}$ 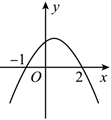 $\text{D.}$
$\text{D.}$ 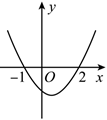
已知函数 $f(x)=(a x-1)(x+b)$, 如果不等式 $f(x)>0$ 的解集为 $(-1,3)$, 那么不等式 $f(-2 x)>0$ 的解集为
$\text{A.}$ $\left(-\infty,-\frac{3}{2}\right) \cup\left(\frac{1}{2},+\infty\right)$
$\text{B.}$ $\left(-\frac{3}{2}, \frac{1}{2}\right)$
$\text{C.}$ $\left(-\infty,-\frac{1}{2}\right) \cup\left(\frac{3}{2},+\infty\right)$
$\text{D.}$ $\left(-\frac{1}{2}, \frac{3}{2}\right)$
已知函数 $f(x)$ 的定义域为 $B$, 函数 $f(1-3 x)$ 的定义域为 $A=\left[\frac{1}{4}, 1\right]$, 若 $\exists x \in B$, 使得 $a>x^2-x+1$ 成立,则实数 $a$ 的取值范围为
$\text{A.}$ $\left(-\infty, \frac{13}{16}\right)$
$\text{B.}$ $\left(0, \frac{13}{16}\right)$
$\text{C.}$ $\left(\frac{13}{16},+\infty\right)$
$\text{D.}$ $\left(-\frac{13}{16}, \frac{13}{16}\right)$
若 $a, b, c \in R$, 则下列命题正确的是()
$\text{A.}$ 若 $a>b, c>d$, 则 $a-c>b-d$
$\text{B.}$ 若 $a>b$, 则 $\frac{1}{a} < \frac{1}{b}$
$\text{C.}$ 若 $a>b$, 则 $a^{3}>b^{3}$
$\text{D.}$ 若 $a>b$, 则 $a c^{2}>b c^{2}$
函数 $y=3 x+\frac{1}{x-1}(x>1)$ 的最小值是
$\text{A.}$ 4
$\text{B.}$ $2 \sqrt{3}-3$
$\text{C.}$ $2 \sqrt{3}$
$\text{D.}$ $2 \sqrt{3}+3$