一、单选题 （共 33 题，每小题 5 分，共 50 分，每题只有一个选项正确）
我国古代典籍《周易》用“卦”描述万物的变化．每一“重卦”由从下到上排列的 6 个爻组成，爻分为阳爻“——”和阴爻“— —”，如图就是一重卦．在所有重卦中随机取一重卦，则该重卦恰有 3 个阳爻的概率是 （ ）
$\text{A.}$ $5 \over 16$
$\text{B.}$ $11 \over 32$
$\text{C.}$ $21 \over 32$
$\text{D.}$ $11 \over 16$
执行如图所示的程序框图,输出的 s 值为 ( )
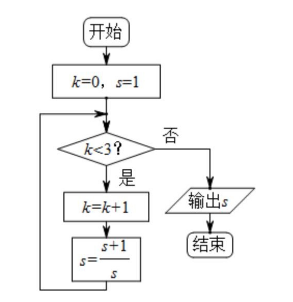
$\text{A.}$ 2
$\text{B.}$ $\frac{3}{2}$
$\text{C.}$ $\frac{5}{3}$
$\text{D.}$ $\frac{8}{5}$
已知 $a=\sin \frac{\pi}{4}, b=e^{\ln \frac{1}{2}}, c=\frac{\pi}{6}$ 执行如图所示的程序框图, 输出的值为
$\text{A.}$ $\frac{1}{2}$
$\text{B.}$ $\frac{\sqrt{2}}{2}$
$\text{C.}$ $\frac{\pi}{6}$
$\text{D.}$ $1$
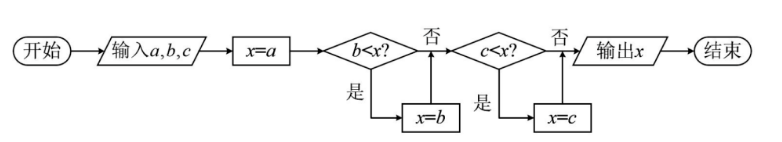
执行如图所示的程序框图, 输出的结果为 258 , 则判断框内可填入的条 件为
$\text{A.}$ $n \geq 4$ ?
$\text{B.}$ $n \geq 5$ ?
$\text{C.}$ $n \geq 6$ ?
$\text{D.}$ $n \geq 7$ ?
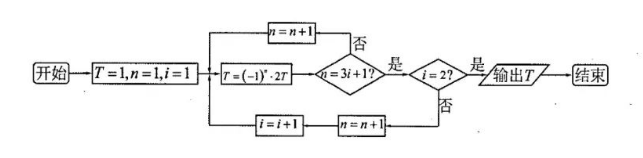
执行如下图所示的程序框图, 则输出的 $T$ 为
$\text{A.}$ $128$
$\text{B.}$ $-32$
$\text{C.}$ $16$
$\text{D.}$ $-64$
如图所示的函数图象与 $x$ 轴均有交点, 其中不能用二分法求图中交点横坐标的是
$\text{A.}$ （1）（3）
$\text{B.}$ （2）（4）
$\text{C.}$ （1）（2）
$\text{D.}$ （3）（4）
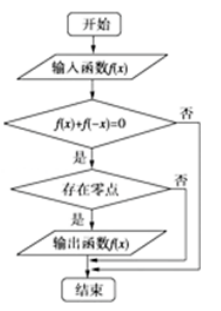
某流程图如右图所示, 现输入如下四个函数, 则可以输出的函数
$\text{A.}$ $f(x)=\frac{2^x-1}{2^x+1}$
$\text{B.}$ $f(x)=\frac{\cos x}{x}\left(-\frac{\pi}{2} < x < \frac{\pi}{2}\right)$
$\text{C.}$ $f(x)=\frac{|x|}{x}$
$\text{D.}$ $f(x)=x^2 \ln \left(x^2+1\right)$
执行如图所示的程序框图，输出的结果是
$\text{A.}$ 56
$\text{B.}$ 54
$\text{C.}$ 36
$\text{D.}$ 64
执行下面的程序框图，输出的 $B=$
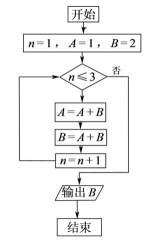
$\text{A.}$ 21
$\text{B.}$ 34
$\text{C.}$ 55
$\text{D.}$ 89
定义有理数复数为实部和虚部均为有理数的复数, 无理数复数为实部和虚部均为无理数的复数, 半有理复数为实部和虚部一个是有理数一个是无理数的复数, 已知在复平面内三角形的三个顶点对应的复数均为半有理数, 则三角形重心对应的复数是
$\text{A.}$ 只能是有理数复数或半有理数复数
$\text{B.}$ 只能是无理数变数或半有理数复数
$\text{C.}$ 只能是半有理数复数
$\text{D.}$ 以上选项均不对
执行下边的程序框图, 输出的 $n=$
$\text{A.}$ 3
$\text{B.}$ 4
$\text{C.}$ 5
$\text{D.}$ 6
已知函数 $g(x)=\frac{x \mathrm{e}^x-x}{\mathrm{e}^x+1}$, 则 $a=g\left(3.1^{3.2}\right), b=g\left(3.2^{3.1}\right), c=g\left(-\pi^{3.2}\right)$ 的大小关系为
$\text{A.}$ $a < b < c$
$\text{B.}$ $c < b < a$
$\text{C.}$ $b < c < a$
$\text{D.}$ $b < a < c$
设 $a=\frac{1}{21}, b=\ln 1.05, c=\mathrm{e}^{0.05}-1$, 则下列关系正确的是
$\text{A.}$ $a>b>c$
$\text{B.}$ $b>a>c$
$\text{C.}$ $c>b>a$
$\text{D.}$ $c>a>b$
若正实数 $a, b$ 满足 $a>b$, 且 $\ln a \cdot \ln b>0$, 则下列不等式一定成立的是
$\text{A.}$ $\log _a b < 0$
$\text{B.}$ $a-\frac{1}{b}>b-\frac{1}{a}$
$\text{C.}$ $2^{a b+1} < 2^{a+b}$
$\text{D.}$ $a^{b-1} < b^{a-1}$
设 $a=0.1 \mathrm{e}^{0.1}, b=\frac{1}{9}, c=-\ln 0.9$, 则
$\text{A.}$ $a < b < c$
$\text{B.}$ $c < b < a$
$\text{C.}$ $c < a < b$
$\text{D.}$ $a < c < b$
设集合 $M=\{2 m-1, m-3\}$, 若 $-3 \in M$, 则实数 $m =$
$\text{A.}$ 0
$\text{B.}$ -1
$\text{C.}$ 0 或 -1
$\text{D.}$ 0 或 1
若集合 $A=\{x \mid x=4 k-3, k \in \mathbf{N}\}, B=\{x \mid(x+3)(x-9) \leq 0\}$, 则 $A \cap B$ 的元素个数为
$\text{A.}$ 2
$\text{B.}$ 3
$\text{C.}$ 4
$\text{D.}$ 5
已知集合 $A=\{x|| x \mid \leq 1\}, B=\{x \mid 2 x-a < 0\}$, 若 $A \subseteq B$, 则实数 $a$ 的取值范围是
$\text{A.}$ $(2,+\infty)$
$\text{B.}$ $[2,+\infty)$
$\text{C.}$ $(-\infty, 2)$
$\text{D.}$ $(-\infty, 2]$
已知集合 $A=\{-1,0,1\}, B=\{x \mid-1 < x < 2\}$, 则 $A \cap B$ 的子集个数为
$\text{A.}$ 1
$\text{B.}$ 2
$\text{C.}$ 3
$\text{D.}$ 4
已知集合 $A=\left\{2,5, m^2-m\right\}, B=\{2, m+3\}$, 若 $A \cap B=B$, 则 $m=$
$\text{A.}$ -3
$\text{B.}$ -1
$\text{C.}$ 2
$\text{D.}$ 3
设集合 $A, B, C$ 均为非空集合, 则下列说法正确的是
$\text{A.}$ 若 $A \cap B=B \cap C$ ，则 $A=C$
$\text{B.}$ 若 $A \cup B=B \cup C$, 则 $A=C$
$\text{C.}$ 若 $A \cap B=B \cup C$, 则 $C \subseteq B$
$\text{D.}$ 若 $A \cup B=B \cap C$, 则 $C \subseteq B$
设集合 $M=\{x \mid x=2 k+1, k \in Z\}, N=\{x \mid x=k+2, k \in Z\}$, 则
$\text{A.}$ $M=N$
$\text{B.}$ $M \subseteq N$
$\text{C.}$ $N \subseteq M$
$\text{D.}$ $M \cap N=\varnothing$
用 $C(A)$ 表示非空集合 $A$ 中的元素个数, 定义 $A * B=\left\{\begin{array}{l}C(A)-C(B), C(A) \geq C(B) \\ C(B)-C(A), C(A) < C(B)\end{array}\right.$ 若 $A=\{1,2\}, B=\left\{x \mid\left(x^2+\right.\right.$ $\left.a x) \cdot\left(x^2+a x+2\right)=0\right\}$, 且 $A * B=1$, 设实数 $a$ 的所有可能取值组成的集合是 $S$, 则 $C(S)$ 等于
$\text{A.}$ 1
$\text{B.}$ 3
$\text{C.}$ 5
$\text{D.}$ 7
已知 $x \in \mathbf{R}$, 则 " $x^3>27$ "是" $|x|>3$ "的
$\text{A.}$ 充分不必要条件
$\text{B.}$ 必要不充分条件
$\text{C.}$ 充要条件
$\text{D.}$ 既不充分也不必要条件
已知直线 $a, b$ 和平面 $\alpha, \beta$, 满足 $a \subset \alpha, b \subset \beta$, 则 " $a$ 和 $b$ 相交" 是 " $a$ 和 $\beta$ 相交" 的
$\text{A.}$ 充分不必要条件
$\text{B.}$ 必要不充分条件
$\text{C.}$ 充要条件
$\text{D.}$ 既不充分也不必要条件
" $m=-2$ " 是 "直线 $l_1: m x+4 y+2=0$ 与直线 $l_2: x+m y+1=0$ 平行" 的
$\text{A.}$ 充要条件
$\text{B.}$ 必要不充分条件
$\text{C.}$ 充分不必要条件
$\text{D.}$ 既不充分也不必要条件
函数 $f(x)=x^3-a x+a-1$ 有两个零点的一个充分不必要条件是
$\text{A.}$ $a=3$
$\text{B.}$ $a=2$
$\text{C.}$ $a=1$
$\text{D.}$ $a=0$
设 $\alpha, \beta$ 均为锐角，则 " $a>2 b$ " 是 " $\sin (\alpha-\beta)>\sin \beta$ " 的
$\text{A.}$ 充分而不必要条件
$\text{B.}$ 必要而不充分条件
$\text{C.}$ 充分必要条件
$\text{D.}$ 既不充分也不必要条件
命题" $\forall x>0, x^2-x+1>0$ "的否定为
$\text{A.}$ $\forall x>0, x^2-x+1 \leq 0$
$\text{B.}$ $\forall x \leq 0, x^2-x+1 \leq 0$
$\text{C.}$ $\exists x>0, x^2-x+1 \leq 0$
$\text{D.}$ $\exists x \leq 0, x^2-x+1 \leq 0$
已知集合 $A=\{x \mid x \geq 0\}$, 集合 $B=\{x \mid x>1\}$, 则以下命题为真命题的是
$\text{A.}$ $\exists x \in A, x \in B$
$\text{B.}$ $\exists x \in B, x \notin A$
$\text{C.}$ $\forall x \in A, x \in B$
$\text{D.}$ $\forall x \in B, x \notin A$
已知命题 $p: \exists x \in \mathbf{R}, \sin x < 1$; 命题 $q: \forall x \in \mathbf{R}, \mathrm{e}^{|x|} \geq 1$, 则下列命题中为真命题的是
$\text{A.}$ $p \wedge q$
$\text{B.}$ $\neg p \wedge q$
$\text{C.}$ $p \wedge \neg q$
$\text{D.}$ $\neg(p \vee q)$
若命题 " $\forall x \in[1,4], x^2-4 x-m \neq 0$ " 是假命题, 则 $m$ 的取值范围是
$\text{A.}$ $-4 \leqslant m \leqslant-3$
$\text{B.}$ $m < -4$
$\text{C.}$ $-4 \leqslant m \leqslant 0$
$\text{D.}$ $m \geqslant-4$
已知函数 $f(x)=\left\{\begin{array}{c}-\frac{1}{4} x+a, x \leq 2 \\ \log _a x, x>2\end{array}\right.$, 若命题 " $\exists x \in \mathbf{R}, f(x) < 1$ "为假命题, 则实数 $a$ 的取值范围是
$\text{A.}$ $\left[\frac{3}{2}, 2\right]$
$\text{B.}$ $(1,2]$
$\text{C.}$ $(1, \sqrt{2}]$
$\text{D.}$ $\left[\sqrt{2}, \frac{3}{2}\right]$
二、多选题 （共 14 题，每小题 5 分，共 20 分, 每题有多个选项符合要求，全部选对得 5 分，部分选对得 2 分，有选错得 0 分）
对正整数 $N$, 若其不能被任意一个完全平方数整除, 则称其为 “无平方因子数”, 并记其的素因子个数为 $d_n$. 由所有 “无平方因子数” 构成的集合记作 $S$. 则数论函数 “缪比乌斯函数” 定义如下
$$
\mu(n)=\left\{\begin{array}{c}
1, n=1 \\
(-1)^{d_n}, n \in S \\
0, n \notin S
\end{array}\right.
$$
则下列运算正确的有
$\text{A.}$ $\mu(1)+\mu(2)=0$
$\text{B.}$ $\mu(1)+\mu(2)+\mu(4)=1$
$\text{C.}$ $\mu(1)+\mu(2)+\mu(4)+\mu(8)=0$
$\text{D.}$ $\mu(1)+\mu(2)+\cdots+\mu\left(2^n\right)=1(n \geq 4)$
已知 $m>n$, 且 $m+n>1$, 则
$\text{A.}$ $2^m>2^n$
$\text{B.}$ $m^2>n^2$
$\text{C.}$ $m^2-m < n^2-n$
$\text{D.}$ $\ln |m|+\ln |n|>0$
若 $a=\ln 1.1, b=\frac{1}{11}, c=\sin 0.1, d=\frac{21}{220}$, 则
$\text{A.}$ $a < b$
$\text{B.}$ $b < c$
$\text{C.}$ $a < d$
$\text{D.}$ $c < d$
已知实数 $a, b$ 满足 $a \mathrm{e}^a=b \ln b=3$, 则
$\text{A.}$ $a=\ln b$
$\text{B.}$ $a b=\mathrm{e}$
$\text{C.}$ $b-a < \mathrm{e}-1$
$\text{D.}$ $\mathrm{e}+1 < a+b < 4$
已知实数 $a, b$, 满足 $a>b>0, \ln a \ln b=1$, 则
$\text{A.}$ $a b>\mathrm{e}^2$
$\text{B.}$ $\log _a 2 < \log _b 2$
$\text{C.}$ $\left(\frac{1}{2}\right)^{a b+1} < \left(\frac{1}{2}\right)^{a+b}$
$\text{D.}$ $a^a b^b>a^b b^a$
已知函数 $f(x)$ 及其导函数 $f^{\prime}(x)$ 的定义域均为 $\mathbf{R}$, 记 $g(x)=f^{\prime}(x)$, 若 $f\left(\frac{3}{2}-2 x\right)$, $g(2+x)$ 均为偶函数, 则
$\text{A.}$ $f(0)=0$
$\text{B.}$ $g\left(-\frac{1}{2}\right)=0$
$\text{C.}$ $f(-1)=f(4)$
$\text{D.}$ $g(-1)=g(2)$
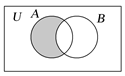
已知全集 $U=\{0,1,2,3,4,5,6,7\}$, 集合 $A=\{x \in \mathbf{N} \mid x < 5\}, B=\{1,3,5,7\}$, 则图中阴影部分所表示的集合为
$\text{A.}$ $\{0,2,4\}$
$\text{B.}$ $\{2,4\}$
$\text{C.}$ $A \cap\left(C_u B\right)$
$\text{D.}$ $((C_u A) \cap(C_u B)$