一、单选题 (共 3 题 ),每题只有一个选项正确
1. 若 , 则 的值是
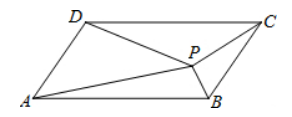
2. 如图, 平行四边形
中,
是四边形内任意一点,
的面积分别为
, 则一定成立的是
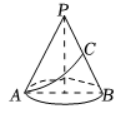
3. 如图,
为圆锥经过底面直径
的最大截面,
, 点
为母线
的中点. 一只蜘蛛要从点
沿圆雉侧面爬到点
, 则该蜘蛛要爬的最短路径长为
9
二、解答题 (共 6 题 ),解答过程应写出必要的文字说明、证明过程或演算步骤
4. 若 , 解方程
5. 在Rt
中,
是
的角平分线,
于点
.在线段
上任取一点
, 以
为一边, 在
的下方作
交
延长线于点
. 请判断线段
之间的数量关系, 并证明你的结论.

6. 在曲线 上点 处作切线, 该切线与曲线以及 轴所围图形的面积为 .
(1) 求点 的坐标和切线方程;
(2) 求由上述平面图形绕 轴旋转一周所成旋转体的体积.
7. 设 , 证明 .
8. 对函数
填写下表:

9. 证明当 时, .