(1) 已知随机变量 $X$ 的概率分布为
$$
P(X=1)=0.2, P(X=2)=0.3, P(X=3)=0.5
$$

试写出 $X$ 的分布函数 $F(x)$.
(2) 求 $X$ 的数学期望与方差.
(3) 已知随机变量 $\boldsymbol{Y}$ 的概率密度为
$$
f(y)= \begin{cases}\frac{y}{a^2} e^{-\frac{y^2}{2 a^2}} & y \geq 0 \\ 0 & y < 0\end{cases}
$$

求随机变量 $Z=\frac{1}{Y}$ 的数学期望 $E(Z)$.

已知某商品的需求量 $D$ 和供给量 $S$ 都是价格 $p$ 的函数:
$$
D=D(p)=\frac{a}{p^2}, S=S(p)=b p ，
$$

其中 $a>0$ 和 $b>0$ 是常数. 价格 $p$ 是时间 $t$ 的函数，且满足方程 $\frac{\mathrm{d} p}{\mathrm{~d} t}=k[D(p)-S(p)],(k$ 是常数 $)$ ，假设当 $t=0$ 时价格为 1 . 试求:
(1) 需求量等于供给量时的均衡价格 $P_e$;
(2) 价格函数 $p(t)$ ；
(3) 极限 $\lim _{t \rightarrow \infty} p(t)$.

Let $G$ be a finite group.
(1) Let $K$ be a field. Show that $G$ has a finite-dimensional fait hful $K$-linear representation.
(2) Show that $G$ has a faithful one-dimensional complex repr esentation if and only if $G$ is cyclic.
(3) Assume moreover that $G$ is commutative. Let $n \geq 1$ be an integer. Show that $G$ has a faithful $n$-dimensional complex re presentation if and only if $G$ can be generated by $n$ elements.
(4) Classify all finite groups having a faithful 2-dimensional re al representation.

Let $n \geq 1$ be an integer and write $\Phi_n(X)$ the $n$-th cyclotomi c polynomial, that is, the minimal polynomial of a primitive $n$ th root of unity in $\mathbb{C}$ over $\mathbb{Q}$. Write also $\varphi(n)=\operatorname{deg}\left(\Phi_n(X)\right)$
(1) Let $q$ be a power of a prime number such that $(q, n)=1$. Show that $\Phi_n$, viewed as an element in $\mathbb{F}_q[X]$, can be decom posed as a product of $\varphi(n) / d$ irreducible polynomials of deg ree $d$, with $d$ the order of $q$ in the multiplicative group $(\mathbb{Z} / n \mathbb{Z})^{\times}$.
(2) From now on, assume $n=2^{r+1}$ for some integer $r \geq 1$. L et $\zeta=\zeta_n$ be a primitive $n$-th root of unity and $K=\mathbb{Q}[\zeta]$. Let $p$ be a prime with $p \equiv-3(\bmod 8)$.
(a) For $x, y \in K=\mathbb{Q}[\zeta]$, define
$$
(x, y):=\sum_\tau \tau(x) \cdot \overline{\tau(y)}
$$
where $\tau$ runs through all the embeddings $K \hookrightarrow \mathbb{C}$ of $K$ into the field $\mathbb{C}$ of complex numbers. Write $K_{\mathbb{R}}=K \otimes_{\mathbb{Q}} \mathbb{R}$, and we use the same notation to denote the (à priori $\mathbb{C}$-valued) bi linear form on $K_{\mathbb{R}}$ obtained by extension of scalars. Show tha $\mathrm{t}(\cdot, \cdot)$ gives an inner product on $K_{\mathbb{R}}$ and for $0 \leq i, j < 2^r$,
$$
\left(\zeta^i, \zeta^j\right)= \begin{cases}2^r, & \text { if } i=j \\ 0, & \text { otherwise }\end{cases}
$$

In particular, we obtain an Euclidean space $K_{\mathbb{R}}$ and $\left(\zeta^i / \sqrt{2^r}\right)_{0 \leq i < 2^r}$ is an orthonormal basis.
(b) Decompose $p \mathcal{O}_K$ into a product of prime ideals.
(c) Let $\mathfrak{p} \subset \mathcal{O}_K$ be a prime ideal of $\mathcal{O}_K$ containing $p$. Show th at for every $\alpha \in \mathfrak{p},|\alpha|^2 \in 2^r p \mathbb{Z}$, and compute the length of $\mathrm{t}$ he shortest non-zero vector in the prime ideal $\mathfrak{p} \subset K_{\mathbb{R}}$.

Consider the integral
$$
\int_0^{\infty} f(x) \mathrm{d} x
$$
where $f$ is continuous, $f^{\prime}(0) \neq 0$, and $f(x)$ decays like $x^{-1-\alpha}$ with $\alpha>0$ in the limit $x \rightarrow \infty$.
(a) Suppose you apply the equispaced composite trapezoid $\mathrm{r}$ ule with $n$ subintervals to approximate
$$
\int_0^L f(x) \mathrm{d} x
$$

What is the asymptotic error formula for the error in the limit $n \rightarrow \infty$ with $L$ fixed?
(b) Suppose you consider the quadrature from (a) to be an ap proximation to the full integral from 0 to $\infty$. How should $L$ in crease with $n$ to optimize the asymptotic rate of total error $d$ ecay? What is the rate of error decrease with this choice of $L$ ?
(c) Make the following change of variable $x=\frac{L(1+y)}{1-y}$, $y=\frac{x-L}{x+L}$ in the original integral to obtain
$$
\int_{-1}^1 F_L(y) \mathrm{d} y
$$

Suppose you apply the equispaced composite trapezoid rule; what is the asymptotic error formula for fixed $L$ ?
(d) Depending on $\alpha$, which method - domain truncation of ch ange-of-variable - is preferable?

Consider the diffusion equation
$$
\frac{\partial v}{\partial t}=\mu \frac{\partial^2 v}{\partial x^2}, \quad v(x, 0)=\phi(x), \quad \int_a^b v(x, t) \mathrm{d} x=0
$$
with $x \in[a, b]$ and periodic boundary conditions. The solutio $\mathrm{n}$ is to be approximated using the central difference operator $L$ for the 1D Laplacian.
$$
L v_m=\frac{v_{m+1}-2 v_m+v_{m-1}}{h^2}
$$
and the following two finite different approximations, (i) Forw ard-Euler
$$
v_{n+1}=v_n+\mu k L v_n,(1)
$$
and (ii) Crank-Nicolson
$$
v_{n+1}=v_n+\mu k\left(L v_n+L v_{n+1}\right)
$$

Throughout, consider $[a, b]=[0,2 \pi]$ and the finite differenc e stencil to have periodic boundary conditions on the spatial lattice $[0, h, 2 h, \cdots,(N-1) h]$ where $h=\frac{2 \pi}{N}$ and $N$ is ev en.
(a) Determine the order of accuracy of the central difference operator $L v$ is approximating the second derivative $v_{x x}$.
(b) Using $v_m^n=\sum_{l=0}^{N-1} \hat{v}_l^n \exp \left(-i \frac{2 \pi l m}{N}\right)$ give the updates $\hat{v}_l^{n+1}$ in terms of $\hat{v}_l^n$ for each of the methods, including the ca se $l=0$.
(c) Give the solution for $v_m^n$ for each method when the initial condition is $\phi(m \Delta x)=(-1)^m$.
(d) What are the stability constraints on the time step $k$ for ea ch of the methods, if any, in equation (1) and (2)? Show there are either no constraints or express them in the form $k \leq F(h, \mu)$

Assume we have $n$ observations: $\left(Y_i, x_i\right), i=1, \cdots, n$, wher e $Y_i$ is the random response and $x_i=\left(x_{i 1}, \cdots, x_{i p}\right)^T$ is a ve ctor of $p$ fixed covariates for the $i$ th observation. Denote $\beta=\left(\beta_1, \cdots, \beta_p\right)$ be a unknown $p$-length vector of regressio n coefficients. Let $\theta_i=\sum_{j=1}^p x_{i j} \beta_j, \mu_i=E\left(Y_i\right)$ and $\sigma_i^2=\operatorname{Var}\left(Y_i\right)$. Assume the density of $Y_i$ belongs to the follo wing exponential family:
$$
f\left(y_i ; \theta_i\right)=\exp \left\{\theta_i y_i-b\left(\theta_i\right)\right\},(1)
$$
where $b^{\prime}\left(\theta_i\right)=\mu_i, b^{\prime \prime}\left(\theta_i\right)=\sigma_i^2$. Suppose that all $\theta_i$ 's are con tained in a compact subset of a space $\Theta$. Let $\ell_n(\beta)$ be the log -likelihood function of the data, and let $H_n(\beta)=-\frac{\partial^2 \ell_n(\beta)}{\partial \beta \partial \beta^T}$.

Let $\mathcal{X}$ be the set of all $p$ covariates under consideration. Let $\alpha_0 \subset \mathcal{X}$ be the subset that contains and only contains all the important covariates affecting $Y$ (the corresponding $\beta_j$ 's are nonzero). Let $\alpha$ be any subset of $\mathcal{X}$, and let $\beta(\alpha)$ be the vect or of the components in $\beta$ that correspond to the covariates $\mathrm{i}$ $\mathrm{n} \alpha$. Let $A=\left\{\alpha: \alpha_0 \subset \alpha\right\}$ be the collection of models that including all important covariates. We assume:
(I) There exist positive constants $C_1, C_2$ such that for all suffic iently large $n$,
$$
C_1 < \lambda_{\min }\left\{\frac{1}{n} H_n(\beta)\right\} < \lambda_{\max }\left\{\frac{1}{n} H_n(\beta)\right\} < C_2
$$
where $\lambda_{\min }\left\{\frac{1}{n} H_n(\beta)\right\}$ and $\lambda_{\max }\left\{\frac{1}{n} H_n(\beta)\right\}$ are the smalles $\mathrm{t}$ and largest eigenvalues of $\frac{1}{n} H_n(\beta)$.
(II) For any given $\varepsilon>0$, there exists a constant $\delta>0$ such th at, when $n$ is sufficiently large,
$$
(1-\varepsilon) H_n(\beta(\alpha)) \leq H_n(\tilde{\beta}) \leq(1+\varepsilon) H_n(\beta(\alpha))
$$
for all $\alpha \in A$ and $\tilde{\beta}$ satisfying $\|\tilde{\beta}-\beta(\alpha)\| \leq \delta$.

For any model $\alpha$. let $\hat{\beta}_\alpha$ be the MLE of $\beta(\alpha)$ based on this m odel. Show that
$$
\max _{\alpha \in A}\left\|\hat{\beta}_\alpha-\beta(\alpha)\right\|=O_p\left(n^{-1 / 3}\right)
$$

已知函数 $f(x)=\left\{\begin{array}{cc}x & 0 \leq x \leq 1 \\ 2-x & 1 < x \leq 2\end{array}\right.$ ，试计算下列各题:
(1) $S_0=\int_0^2 f(x) e^{-x} \mathrm{~d} x$;
(2) $S_1=\int_2^4 f(x-2) e^{-x} \mathrm{~d} x$;
(3) $S_n=\int_{2 n}^{2 n+2} f(x-2 n) e^{-x} \mathrm{~d} x(n=2,3, \cdots)$ ；
(4) $S=\sum_{n=0}^{\infty} S_n$.

已知随机变量 $\boldsymbol{X}$ 和 $Y$ 的联合概率分布为:

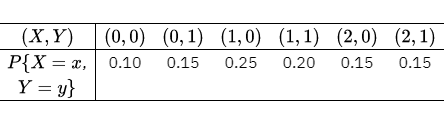
求：(1) $X$ 的概率分布;
(2) $X+Y$ 的概率分布;
(3) $Z=\sin \frac{\pi(X+Y)}{2}$ 的数学期望.

设 $\alpha_1=\left[\begin{array}{c}1+\lambda \\ 1 \\ 1\end{array}\right], \alpha_2=\left[\begin{array}{c}1 \\ 1+\lambda \\ 1\end{array}\right], \alpha_3=\left[\begin{array}{c}1 \\ 1 \\ 1+\lambda\end{array}\right], \beta=\left[\begin{array}{c}0 \\ \lambda \\ \lambda^2\end{array}\right]$,
问 ${\lambda}$ 取何值时，
（1） $\beta$ 可由 $\alpha_1, \alpha_2, \alpha_3$ 线性表示，且表达式
唯一?
（2） $\beta$ 可由 $\alpha_1, \alpha_2, \alpha_3$ 线性表示，且表达式不唯一?
（3）$\beta$ 不能由 $\alpha_1, \alpha_2, \alpha_3$ 线性表示?