数轴
(1) 若
(2) 若
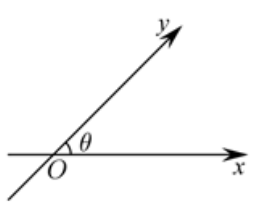
(1) 若
(2) 若
