如图所示, 设
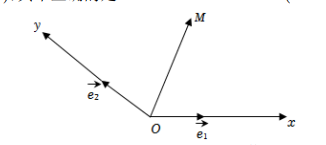

A.