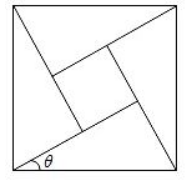
公元三世纪,我国汉代数学家赵爽在注解 《周髀算经》时给出的“赵爽弦图”如图所示,它是由四个全等 的直角三角形与中间的小正方形拼成的一个大正方形. 如果大正方形的面积是 125 ,小正方形面积是 25 ,则 $(\sin \theta+\cos \theta)^2= $
$\text{A.}$ $\frac{9}{5}$
$\text{B.}$ $\frac{\sqrt{5}}{5}$
$\text{C.}$ $\frac{3 \sqrt{5}}{5}$
$\text{D.}$ $\frac{1}{5}$