如图, 在菱形 $A B C D$ 中, 对角线 $A C 、 B D$ 交于点 $O$, 以 $A C$ 为斜边作 $R t \triangle A E C, A E$ 与 $B D$ 交于点 $F$, 连接 $B E$, 使得 $B F=C O$, 且 $\angle E B F=2 \angle C A E$, 若 $A C=2$, 则菱形 $A B C D$ 的周长为
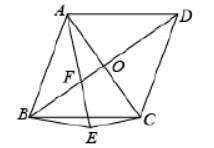
$\text{A.}$ $4 \sqrt{6}$
$\text{B.}$ $4 \sqrt{3}$
$\text{C.}$ $4 \sqrt{2}$
$\text{D.}$ $4$