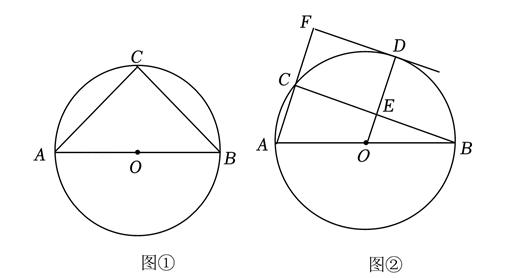
已知 $A B$ 为 $\odot O$ 的直径, $A B=8, C$ 为 $\odot O$ 上一点, 连接 $C A, C B$.
(I) 如图(1), 若 $C$ 为 $A B$ 的中点, 求 $\angle C A B$ 的大小和 $A C$ 的长;
(II) 如图(2), 若 $A C=2, O D$ 为 $\odot O$ 的半径, 且 $O D \perp C B$, 垂足为 $E$, 过点 $D$ 作 $\odot O$ 的 切线, 与 $A C$ 的延长线相交于点 $F$, 求 $F D$ 的长.