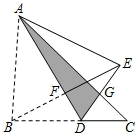
重庆市2020年初中学业水平暨高中招生考试-Kmath科数- 中考、高考、考验数学题库

.如图, 三角形纸片 $A B C$, 点 $D$ 是 $B C$ 边上一点, 连接 $A D$, 把 $\triangle A B D$ 沿着 $A D$ 翻折, 得到 $V A E D, D E$ 与 $\mathrm{AC}$ 交于点 $G$, 连接 $B E$ 交 $A D$ 于点 $F$. 若 $D G=G E, A F=3, B F=2, \mathrm{~V} A D G$ 的面积为 2 , 则点 $F$ 到 $B C$ 的距离为

$ \text{A.}$ $\frac{\sqrt{5}}{5}$ $ \text{B.}$ $\frac{2 \sqrt{5}}{5}$ $ \text{C.}$ $\frac{4 \sqrt{5}}{5}$ $ \text{D.}$ $\frac{4 \sqrt{3}}{3}$

0 人点赞

22 次查看

我来讲解

答案：

解析：

【详解】解: $\because D G=G E$,
$$
\begin{aligned}
& \therefore S_{\triangle A D G}=S_{\triangle A E G}=2, \\
& \therefore S_{\triangle A D E}=4,
\end{aligned}
$$
由翻折可知, $\mathrm{V}_{A D} \cong \mathrm{V}_{A D E}, B E \perp A D$,
$$
\begin{aligned}
& \therefore S_{\triangle A B D}=S_{\triangle A D E}=4, \angle B F D=90^{\circ}, \\
& \therefore \frac{1}{2} \cdot(A F+D F) \cdot B F=4, \\
& \therefore \frac{1}{2} \cdot(3+D F) \cdot 2=4, \\
& \therefore D F=1, \\
& \therefore D B=\sqrt{B F^2+D F^2}=\sqrt{1^2+2^2}=\sqrt{5},
\end{aligned}
$$
设点 $F$ 到 $B D$ 的距离为 $h$,
则 $\frac{1}{2} \cdot B D \cdot h=\frac{1}{2} \cdot B F \cdot D F$,
$$
\therefore h=\frac{2 \sqrt{5}}{5} \text {, }
$$
故选: $B$.

①点击首页查看更多试卷和试题 , 点击查看本题所在试卷
② 下载本题Word版或下载本题PDF版点击赞助本站

关闭