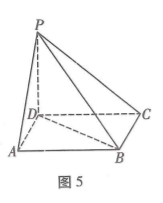
如图 5, 在四棱雉 $P-A B C D$ 中, 底面 $A B C D$ 是平行四边形, $P D \perp$ 平面 $A B C D, P D=A D=B D=1, A B=\sqrt{2}$.
(1) 求证: 平面 $P B D \perp$ 平面 $P B C$;
(2) 试问在线段 $P C$ 上是否存在一点 $M$, 使得二面角 $M-B D-C$ 的大小为 $60^{\circ}$, 若存在求出 $\frac{P M}{M C}$ 的值; 若不存在, 请说明理由.
$\text{A.}$
$\text{B.}$
$\text{C.}$
$\text{D.}$